| (One intermediate revision by one other user not shown) | |||
| Line 1: | Line 1: | ||
| − | + | [[Category:ECE302Fall2008_ProfSanghavi]] | |
| + | [[Category:probabilities]] | ||
| + | [[Category:ECE302]] | ||
| + | [[Category:problem solving]] | ||
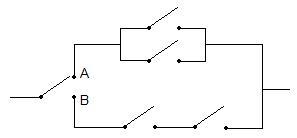
| − | '''The Answer:''' | + | '''The Problem:''' What is the probability each circuit will be connected? The probability each switch will be connected is <math>p\!</math> and <math>P(A)=\frac{2}{3}</math> and <math>P(B)=\frac{1}{3}</math>.<br> |
| + | |||
| + | '''The Answer:'''<br> | ||
[[Image:Sw1_ECE302Fall2008sanghavi.jpg]] | [[Image:Sw1_ECE302Fall2008sanghavi.jpg]] | ||
| − | + | <br> | |
| + | The probablity of the top path (path <math>A\!</math>) being connected is <math>1-(1-p)^2\!</math>, which is found by finding the probability the path won't be connected and subtracting it from 1. The probability of the bottom path (path <math>B\!</math>) is obviously <math>p^2\!</math>. Thus <br><br> | ||
| + | <math>P(OC)=\frac{2}{3}[1-(1-p)^2]+\frac{1}{3}p^2</math> | ||
== == | == == | ||
[[Image:Sw2_ECE302Fall2008sanghavi.jpg]] | [[Image:Sw2_ECE302Fall2008sanghavi.jpg]] | ||
| + | |||
| + | <br> | ||
| + | For this design note it is simply the previous problem with another option added in the middle. Thus we can say<br><br> | ||
| + | <math>P(OC)=P(OC|MC)P(MC)+P(OC|MNC)P(MNC)\!</math> where | ||
| + | |||
| + | <br><math>OC = \!</math> Overall Connected<br><math>MC = \!</math> Middle Connected<br><math>MNC = \!</math> Middle Not Connected | ||
| + | <br><br> | ||
| + | and | ||
| + | <br> | ||
| + | <br> | ||
| + | <math>P(OC|MC)\!</math> is <math>1\!</math> (if the middle is connected then the entire circuit is!)<br> | ||
| + | <math>P(MC)\!</math> is just <math>p\!</math> (probability of the middle switch being connected)<br> | ||
| + | <math>P(OC|MNC)\!</math> is simply the answer from the first part<br> | ||
| + | <math>P(MNC)\!</math> is one minus the probability the middle is connected, so <math>1-p\!</math><br> | ||
| + | <br> | ||
| + | Thus: | ||
| + | <br><math>P(OC)=1p + (1-p)[\frac{2}{3}[1-(1-p)^2]+\frac{1}{3}p^2]\!</math> | ||
| + | ---- | ||
| + | [[Main_Page_ECE302Fall2008sanghavi|Back to ECE302 Fall 2008 Prof. Sanghavi]] | ||
Latest revision as of 13:16, 22 November 2011
The Problem: What is the probability each circuit will be connected? The probability each switch will be connected is $ p\! $ and $ P(A)=\frac{2}{3} $ and $ P(B)=\frac{1}{3} $.
The probablity of the top path (path $ A\! $) being connected is $ 1-(1-p)^2\! $, which is found by finding the probability the path won't be connected and subtracting it from 1. The probability of the bottom path (path $ B\! $) is obviously $ p^2\! $. Thus
$ P(OC)=\frac{2}{3}[1-(1-p)^2]+\frac{1}{3}p^2 $
For this design note it is simply the previous problem with another option added in the middle. Thus we can say
$ P(OC)=P(OC|MC)P(MC)+P(OC|MNC)P(MNC)\! $ where
$ OC = \! $ Overall Connected
$ MC = \! $ Middle Connected
$ MNC = \! $ Middle Not Connected
and
$ P(OC|MC)\! $ is $ 1\! $ (if the middle is connected then the entire circuit is!)
$ P(MC)\! $ is just $ p\! $ (probability of the middle switch being connected)
$ P(OC|MNC)\! $ is simply the answer from the first part
$ P(MNC)\! $ is one minus the probability the middle is connected, so $ 1-p\! $
Thus:
$ P(OC)=1p + (1-p)[\frac{2}{3}[1-(1-p)^2]+\frac{1}{3}p^2]\! $