| (14 intermediate revisions by one other user not shown) | |||
| Line 1: | Line 1: | ||
| − | + | [[Category:ECE438 (BoutinFall2009)]] | |
| − | |||
| − | + | == '''Rep Function:''' ( Class Notes from 26/08/09, [[ECE438|ECE 438]], [[user:mboutin|Prof Boutin]])== | |
| − | + | A rep function periodically repeats another function with some specified period T ( basically sampling time). Mathematically a rep operator is a function x(t) convolved with a summation of shifted deltas: | |
| − | + | <math> rep_T [x(t)] = x(t)* \mathrm {P_T}(t) = x(t)* \sum_{k=-\infty}^{\infty}\delta(t-kT) </math> | |
| − | + | <math> {{=}}\sum_{\infty}^{\infty}x(\delta(t-kT) </math> | |
| + | |||
| + | <math> {{=}}\sum_{k=-\infty}^{\infty}x(t-kT) </math> | ||
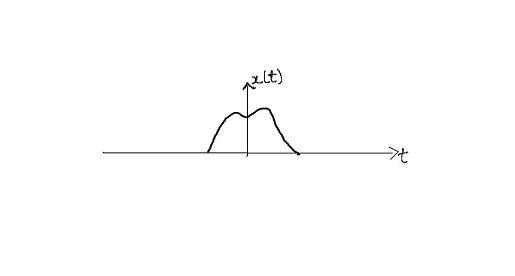
If below is the x(t): | If below is the x(t): | ||
| Line 15: | Line 16: | ||
[[Image:functiona.jpg]] | [[Image:functiona.jpg]] | ||
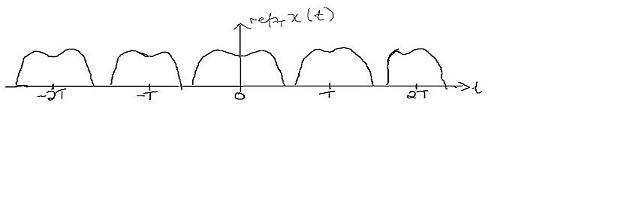
| − | Then the <math>rep_T | + | Then the <math>rep_T [x(t)] </math> looks like: |
| − | [[Image: | + | [[Image:reppeda.jpg]] |
The resulting function is periodic with period T. To keep x(t) repeating without aliasing, the minimum sampling period should be <math> \geqq 2 * T </math> | The resulting function is periodic with period T. To keep x(t) repeating without aliasing, the minimum sampling period should be <math> \geqq 2 * T </math> | ||
| − | '''Comb Function''' | + | |
| + | == '''Comb Function''' == | ||
| + | |||
A comb function multiplies a function with a periodic train of impulses. | A comb function multiplies a function with a periodic train of impulses. | ||
| − | <math> comb_T | + | <math> comb_T[x(t)] = x(t) . \sum_{k=-\infty}^{\infty}\delta(t-kT) = x(t) . \mathrm{P_T} (t) </math> |
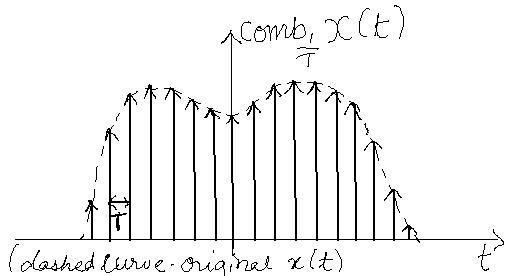
Using the same x(t) as above as our function to be multiplied with a train of impulses, we get: | Using the same x(t) as above as our function to be multiplied with a train of impulses, we get: | ||
| − | [[Image:]] | + | [[Image:combed.jpg]] |
| + | |||
'''Note''': The Fourier Transform of a <math> rep_T </math> x(t) gives the comb function of x(t) but in the frequency domain. | '''Note''': The Fourier Transform of a <math> rep_T </math> x(t) gives the comb function of x(t) but in the frequency domain. | ||
| Line 39: | Line 43: | ||
<math> \mathrm{P_T}(f) = \mathfrak{F} ( \sum_{k} \delta(t-kT)) </math> | <math> \mathrm{P_T}(f) = \mathfrak{F} ( \sum_{k} \delta(t-kT)) </math> | ||
| − | <math> {{=}} \mathfrak{F} ( \sum_{n=-\infty}^{\infty} \ | + | <math> {{=}} \mathfrak{F} (\sum_{n=-\infty}^{\infty} \frac{1}{T} exp \frac{j2nt\pi}{T} ) </math> |
| + | |||
| + | <math> {{=}} \sum_{n=-\infty}^{\infty} \frac{1}{T} \delta( f - \frac{n}{T} ) </math> | ||
| + | |||
| + | <math> {{=}} \frac{1}{T} P_T (f) </math> | ||
| + | |||
| + | Therefore: | ||
| + | |||
| + | <math> \mathrm{X}(f).\frac{1}{T}P_T (f) {{=}} \frac{1}{T}comb_\frac{1}{T} [ \mathrm{X}(f)] </math> | ||
| + | |||
| + | To conclude the transform relationship between the comb operator and rep operator surfaces when we take the operator's Continuous Time Fourier Transform : | ||
| + | |||
| + | <math> comb_T [x(t)] \iff \frac{1}{T}rep_\frac{1}{T} [ \mathrm{X}(f)] </math> | ||
| + | |||
| + | <math> rep_T [x(t)] \iff \frac{1}{T}comb_\frac{1}{T} [ \mathrm{X}(f)] </math> | ||
| + | ---- | ||
| + | [[ ECE438 (BoutinFall2009)|Back to ECE438 (BoutinFall2009)]] | ||
Latest revision as of 07:38, 25 August 2010
Rep Function: ( Class Notes from 26/08/09, ECE 438, Prof Boutin)
A rep function periodically repeats another function with some specified period T ( basically sampling time). Mathematically a rep operator is a function x(t) convolved with a summation of shifted deltas:
$ rep_T [x(t)] = x(t)* \mathrm {P_T}(t) = x(t)* \sum_{k=-\infty}^{\infty}\delta(t-kT) $
$ {{=}}\sum_{\infty}^{\infty}x(\delta(t-kT) $ $ {{=}}\sum_{k=-\infty}^{\infty}x(t-kT) $
If below is the x(t):
Then the $ rep_T [x(t)] $ looks like:
The resulting function is periodic with period T. To keep x(t) repeating without aliasing, the minimum sampling period should be $ \geqq 2 * T $
Comb Function
A comb function multiplies a function with a periodic train of impulses.
$ comb_T[x(t)] = x(t) . \sum_{k=-\infty}^{\infty}\delta(t-kT) = x(t) . \mathrm{P_T} (t) $
Using the same x(t) as above as our function to be multiplied with a train of impulses, we get:
Note: The Fourier Transform of a $ rep_T $ x(t) gives the comb function of x(t) but in the frequency domain.
$ \mathfrak{F} (rep_T [x(t)]) = \mathfrak{F} ( x(t) * P_T (t) ) = \mathrm{X}(f).\mathrm{P_T}(f) $
Where
$ \mathrm{P_T}(f) = \mathfrak{F} ( \sum_{k} \delta(t-kT)) $
$ {{=}} \mathfrak{F} (\sum_{n=-\infty}^{\infty} \frac{1}{T} exp \frac{j2nt\pi}{T} ) $ $ {{=}} \sum_{n=-\infty}^{\infty} \frac{1}{T} \delta( f - \frac{n}{T} ) $ $ {{=}} \frac{1}{T} P_T (f) $
Therefore:
$ \mathrm{X}(f).\frac{1}{T}P_T (f) {{=}} \frac{1}{T}comb_\frac{1}{T} [ \mathrm{X}(f)] $
To conclude the transform relationship between the comb operator and rep operator surfaces when we take the operator's Continuous Time Fourier Transform :
$ comb_T [x(t)] \iff \frac{1}{T}rep_\frac{1}{T} [ \mathrm{X}(f)] $
$ rep_T [x(t)] \iff \frac{1}{T}comb_\frac{1}{T} [ \mathrm{X}(f)] $