| (16 intermediate revisions by 2 users not shown) | |||
| Line 5: | Line 5: | ||
[[Category:signal processing]] | [[Category:signal processing]] | ||
| − | <center><font size= | + | <center> |
| + | <font size= 4> | ||
Topic 3:Fourier transform of "rep" and "comb" | Topic 3:Fourier transform of "rep" and "comb" | ||
</font size> | </font size> | ||
| Line 15: | Line 16: | ||
---- | ---- | ||
---- | ---- | ||
| − | |||
| + | ==1.INTRODUCTION:== | ||
| + | The topic 3 is the Fourier Transform of the Comb and Rep function. In my selecture, I am going to introduce the definition, the Fourier Transformation and the relationship of Comb function and Rep function. | ||
| − | + | ==2.THEORY:== | |
| − | + | (1) | |
| − | 1 | + | |
| − | + | ||
| − | + | ||
| − | + | [[Image:Combfunction3.jpg]] | |
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | |||
Reference:https://engineering.purdue.edu/~bouman/ece637/notes/pdf/RepComb.pdf | Reference:https://engineering.purdue.edu/~bouman/ece637/notes/pdf/RepComb.pdf | ||
| − | <font size= | + | <font size= 4>According to the definition of the comb function: </font size> |
| Line 41: | Line 35: | ||
| − | <font size= | + | <font size= 4> where</font size> <math>P_T(t)= \sum_{n=-\infty}^\infty \delta(t-nT)</math> |
| − | <font size= | + | <font size= 4>Do the Fourier Transform to the function:</font size> |
| Line 50: | Line 44: | ||
| − | <font size= | + | <font size= 4> |
According to the property of Fourier Transformation, the multiplication in the time domain is equal to the convolution in the frequency domain.</font size> | According to the property of Fourier Transformation, the multiplication in the time domain is equal to the convolution in the frequency domain.</font size> | ||
| Line 57: | Line 51: | ||
<math>=x(f)*F\big(P_T(t)\big)</math> | <math>=x(f)*F\big(P_T(t)\big)</math> | ||
| − | <font size= | + | <font size= 4>Because </font size> <math>P_T(t)= \sum_{n=-\infty}^\infty \delta(t-nT)</math> <font size= 4> is a periodic function , so we can expand it to Fourier series. </font size> |
| Line 67: | Line 61: | ||
<math>=\frac{1}{T}</math> | <math>=\frac{1}{T}</math> | ||
| − | <font size= | + | <font size= 4>So, </font size> <math>P_T(t) = \frac{1}{T}\sum_{n=-\infty}^\infty F_n e^{jn\cdot 2\pi t/T} </math> |
<math>=\sum_{n=-\infty}^\infty \frac{1}{T} F(e^{jn\cdot 2\pi t/T}) </math> | <math>=\sum_{n=-\infty}^\infty \frac{1}{T} F(e^{jn\cdot 2\pi t/T}) </math> | ||
| Line 75: | Line 69: | ||
<math>= \frac{1}{T}P_{1/T}(f)</math> | <math>= \frac{1}{T}P_{1/T}(f)</math> | ||
| − | <font size= | + | <font size= 4>So, </font size> <math>F\bigg(comb_T\big(x(t)\big)\bigg)=X(f)*\frac{1}{T}P_{1/T}(f)</math> |
<math>=\frac{1}{T}X(f)*P_{1/T}(f)</math> | <math>=\frac{1}{T}X(f)*P_{1/T}(f)</math> | ||
| Line 81: | Line 75: | ||
<math>=\frac{1}{T}rep_{1/T}X(f)</math> | <math>=\frac{1}{T}rep_{1/T}X(f)</math> | ||
| + | ---- | ||
| + | <font size =4>(2)</font size> | ||
| + | |||
| + | [[Image:Repfunction3.jpg]] | ||
| − | |||
Reference:https://engineering.purdue.edu/~bouman/ece637/notes/pdf/RepComb.pdf | Reference:https://engineering.purdue.edu/~bouman/ece637/notes/pdf/RepComb.pdf | ||
| − | <font size= | + | <font size= 4>According to the definition of Rep function:</font size> |
| Line 97: | Line 94: | ||
| − | <font size= | + | <font size= 4>Use the impluse-train we get previously, according to the conclusion we get from Fourier Transformation of it, we know:</font size> |
<math>F\big(P_T(t)\big)=\frac{1}{T}P_{1/T}(f)</math> | <math>F\big(P_T(t)\big)=\frac{1}{T}P_{1/T}(f)</math> | ||
| − | <font size= | + | <font size= 4>So, </font size> <math>F\bigg(rep_T\big(x(t)\big)\bigg)=x(f)\cdot\frac{1}{T}P_{1/T}(f)</math> |
<math>=\frac{1}{T}x(f)\cdot P_{1/T}(f)</math> | <math>=\frac{1}{T}x(f)\cdot P_{1/T}(f)</math> | ||
| − | |||
| − | |||
==[[slecture_title_of_slecture_review|Questions and comments]]== | ==[[slecture_title_of_slecture_review|Questions and comments]]== | ||
If you have any questions, comments, etc. please post them on [[slecture_title_of_slecture_review|this page]]. | If you have any questions, comments, etc. please post them on [[slecture_title_of_slecture_review|this page]]. | ||
---- | ---- | ||
| − | [[ | + | [[2014_Fall_ECE_438_Boutin_digital_signal_processing_slectures|Back to ECE438 slectures, Fall 2014]] |
Latest revision as of 18:55, 16 March 2015
Topic 3:Fourier transform of "rep" and "comb"
A slecture by ECE student Youqin Liu
Partly based on the ECE438 Fall 2014 lecture material of Prof. Mireille Boutin.
1.INTRODUCTION:
The topic 3 is the Fourier Transform of the Comb and Rep function. In my selecture, I am going to introduce the definition, the Fourier Transformation and the relationship of Comb function and Rep function.
2.THEORY:
(1)
Reference:https://engineering.purdue.edu/~bouman/ece637/notes/pdf/RepComb.pdf
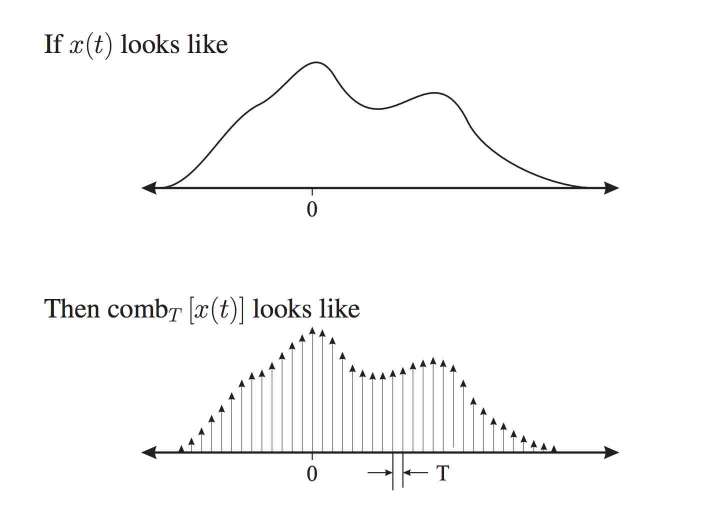
According to the definition of the comb function:
$ comb_T\big(X(t)\big)= x(t)\cdot\ P_T(t) $
where $ P_T(t)= \sum_{n=-\infty}^\infty \delta(t-nT) $
Do the Fourier Transform to the function:
$ F\bigg(comb_T\big(x(t)\big)\bigg) = F\big(x(t)\cdot P_T(t)\big) $
According to the property of Fourier Transformation, the multiplication in the time domain is equal to the convolution in the frequency domain.
$ F\bigg(comb_T\big(x(t)\big)\bigg) = F\big(x(t)\big)* F\big(P_T(t)\big) $
$ =x(f)*F\big(P_T(t)\big) $
Because $ P_T(t)= \sum_{n=-\infty}^\infty \delta(t-nT) $ is a periodic function , so we can expand it to Fourier series.
$ P_T(t)=\sum_{n=-\infty}^\infty F_n e^{jn\cdot 2\pi t/T} $
$ \Rightarrow F_n = \frac{1}{T}\int\limits_{-T/2}^{T/2}P_T(t)e^{jn\cdot 2\pi t/T}dt $
$ =\frac{1}{T} $
So, $ P_T(t) = \frac{1}{T}\sum_{n=-\infty}^\infty F_n e^{jn\cdot 2\pi t/T} $
$ =\sum_{n=-\infty}^\infty \frac{1}{T} F(e^{jn\cdot 2\pi t/T}) $
$ =\sum_{n=-\infty}^\infty \frac{1}{T} \delta(f-\frac{n}{T}) $
$ = \frac{1}{T}P_{1/T}(f) $
So, $ F\bigg(comb_T\big(x(t)\big)\bigg)=X(f)*\frac{1}{T}P_{1/T}(f) $
$ =\frac{1}{T}X(f)*P_{1/T}(f) $ $ =\frac{1}{T}rep_{1/T}X(f) $
(2)
Reference:https://engineering.purdue.edu/~bouman/ece637/notes/pdf/RepComb.pdf
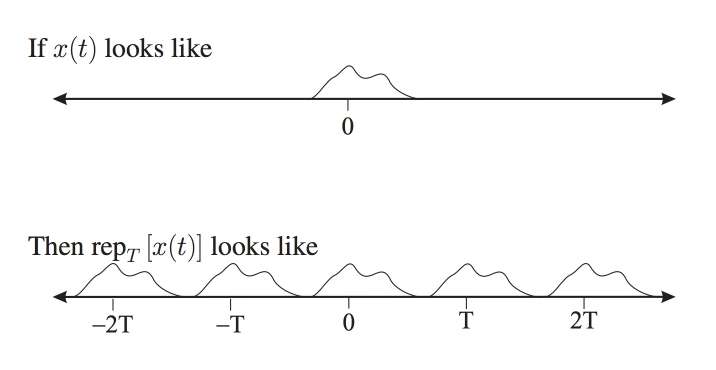
According to the definition of Rep function:
$ rep_T\big(x(t)\big):= x(t)*P_T(t) $
$ =x(t)*\sum_{n=-\infty}^\infty \delta(t-nT) $
So, $ F\bigg(rep_T\big(x(t)\big)\bigg)=F\bigg(x(t)*\sum_{n=-\infty}^\infty \delta(t-nT)\bigg) $
Use the impluse-train we get previously, according to the conclusion we get from Fourier Transformation of it, we know:
$ F\big(P_T(t)\big)=\frac{1}{T}P_{1/T}(f) $
So, $ F\bigg(rep_T\big(x(t)\big)\bigg)=x(f)\cdot\frac{1}{T}P_{1/T}(f) $
$ =\frac{1}{T}x(f)\cdot P_{1/T}(f) $
Questions and comments
If you have any questions, comments, etc. please post them on this page.