Thévenin's theorem
by ECET undergraduate student Justin Flick.
The application of Thévenin's theorem enables multiple voltage and/or current sources and resistors to be represented by a single voltge source and resistor. This greatly simplifies analysis and troubleshooting a circuit when changes are made in areas other than the power supply.
One set of rules to 'Thévenize' a circuit is as follows.
- Find the unloaded voltage output of the source using voltage divider rules.
- Find the total resistance of the circuit across the nodes 'connected to the outside'. This can be done by replacing all voltage sources with a short all current sources with an open circuit.
Example:
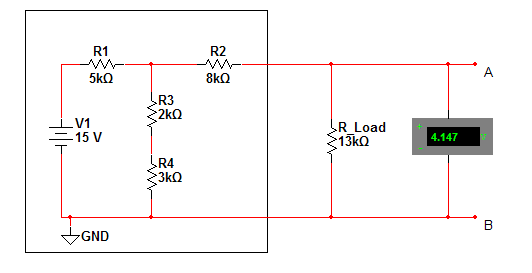
A 15Vdc source is connected to a 4 resistor series parallel circuit with output nodes A and B.
Step 1. Use voltage divider rules to find the unloaded voltage at the output.
$ V_{out}=15V*\frac{5k\Omega}{(2k\Omega+3k\Omega)}=7.5V $
$ R_{total} = 8k\Omega + \frac{1}{(2k\Omega+3k\Omega)^{-1}+ 5k\Omega^{-1}} = 10.5k\Omega $
Verification:
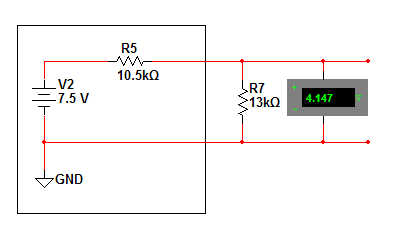
Testing a Thevenin power supply under load should reveal that it is equivilent to the original. placing a 13kohm load in parallel with the output will load both circuits similarly.
$ 15V_{Ein}-\frac{15V_{Ein}*5k\Omega_{load}}{((2k\Omega_{R3}+3k\Omega_{R4})^{-1}+(8k\Omega_{R2}+13k\Omega_{Rload})^{-1})^{-1}+5) }*\frac{13k\Omega_{R1}}{(8k\Omega_{R2}+13k\Omega_{Rload})}=4.148V $
$ 7.5V*\frac{13k\Omega}{(10.5k\Omega+13k\Omega)}=4.148V $