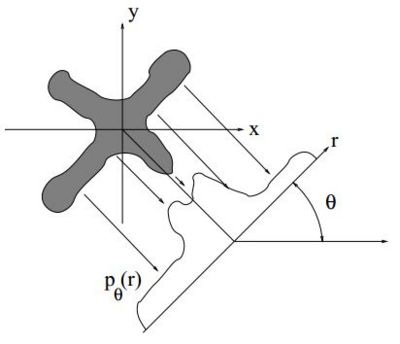
| Line 86: | Line 86: | ||
*Large superconducting magnet | *Large superconducting magnet | ||
** Uniform field within bore | ** Uniform field within bore | ||
| − | ** Very large static magnetic field | + | ** Very large static magnetic field <math>M_0</math> |
| + | |||
| + | |||
| + | ---- | ||
| + | |||
| + | ==Magnetic Field Gradients== | ||
| + | |||
| + | * Magnetic field magnitude at the location <math>(x,y,z)</math> has the form <br/> | ||
| + | <math>M(x,y,z) = M_0 + xG_x + yG_y + zG_z \ </math> <br/> | ||
| + | - <math>G_x</math>, <math>G_y</math> and <math>G_z</math> control magnetic field gradients <br/> | ||
| + | - Gradients can be changed with time <br/> | ||
| + | - Gradients are small compared to <math>M_0</math> | ||
| + | |||
| + | * For the time varying gradients, | ||
| + | <math>M(x,y,z,t) = M_0 + xG_x(t) + yG_y(t) + zG_z(t) \ </math> | ||
| + | |||
| + | |||
| + | ---- | ||
| + | |||
| + | ==MRI Slice Select== | ||
| + | |||
| + | [[Image:intro_fig4.jpeg|400px|thumb|left|Fig 5: MRI slice select]] | ||
| + | |||
| + | Design RF pulse to excite protons in single slice <br/> | ||
| + | - Turn off <math>x</math> and <math>y</math> gradients <br/> | ||
| + | - Set <math>z</math> gradient to fix positive value, <math>G_z > 0</math> <br/> | ||
| + | - Use the fact that resonance frequency is given by <br/> | ||
| + | <math>\omega = L(M_0+zG_z)</math> | ||
| + | |||
| + | |||
| + | ---- | ||
| + | |||
| + | ==Slice Select Pulse Design== | ||
| + | |||
| + | *Design parameters | ||
| + | **Slice center<math> = z_c</math> | ||
| + | **Slice thickness <math> = \Delta z</math> | ||
| + | |||
| + | *Slice centered at <math>z_c</math> ⇒ pulse frequency <br/> | ||
| + | <math> f_c = \frac{LM_0}{2\pi}+\frac{z_cLG_z}{2\pi} = f_0 + \frac{z_cLG_z}{2\pi}</math> | ||
| + | |||
| + | * Slice thickness <math>\Delta z</math> ⇒ pulse bandwidth <br/> | ||
| + | <math>\Delta f = \frac{\Delta z LG_z}{2\pi}</math> | ||
| + | |||
| + | * Using the parameters. the pulse is given by <br/> | ||
| + | <math>s(t) = e^{j2\pi f_ct}sinc(t\Delta f)</math> | ||
| + | |||
| + | and its CTFT is given by <br/> | ||
| + | <math>S(f) = rect(\frac{f-f_c}{\Delta f})</math> | ||
| + | |||
| + | |||
| + | ---- | ||
| + | |||
| + | ==Imaging the Selected Slice == | ||
| + | |||
| + | [[Image:intro_fig4.jpeg|400px|thumb|left|Fig 6:]] | ||
| + | |||
| + | * Precessing atoms radiate electromagnetic energy at RF frequencies | ||
| + | *Strategy | ||
| + | **Vary magnetic gradients along <math>x</math> and <math>y</math> axes | ||
| + | **Measure received RF signal | ||
| + | **Reconstruct image from RF measurements | ||
| + | |||
| + | |||
| + | ---- | ||
| + | |||
| + | ==Signal from a Single Voxel== | ||
| + | |||
| + | [[Image:intro_fig4.jpeg|400px|thumb|left|Fig 6: Signal from a single voxel]] | ||
| + | |||
| + | |||
| + | RF signal from a single voxel has the form <br/> | ||
| + | <math>r(x,y,t) = f(x,y)e^{j\phi(t)} \ </math> | ||
| + | |||
| + | <math>f(x,y)</math> voxel dependent weight | ||
| + | *Depends on properties of material in voxel | ||
| + | *Quantity of interest | ||
| + | *Typically "weighted" by T1, T2, or T3* | ||
| + | |||
| + | <math>\phi(t)</math> phase of received signal | ||
| + | *Can be modulated using <math>G_x</math> and <math>G_y</math> magnetic field gradients | ||
| + | * We assume that <math>\phi(0) = 0</math> | ||
| + | |||
| + | |||
| + | ---- | ||
| + | |||
| + | ==Analysis of Phase== | ||
| + | |||
| + | Frequency = time derivative of phase <br/> | ||
| + | <math>\begin{align} | ||
| + | \frac{d\phi(t)}{dt} &= LM(x,y,t) \\ | ||
| + | \phi(t) &= \int_0^t LM(x,y,\tau)d\tau \\ | ||
| + | &= \int_0^t LM_0 + xLG_x(\tau) + yLG_y(\tau)d\tau \\ | ||
| + | &= \omega_0t + xk_x(t) +yky(t) | ||
| + | \end{align}</math> | ||
| + | |||
| + | where we define <br/> | ||
| + | <math>\omega_0 = LM_0</math> <br/> | ||
| + | <math>k_x(t) = \int_0^t LG_x(\tau)d\tau</math> <br/> | ||
| + | <math>k_y(t) = \int_0^t LG_y(\tau)d\tau</math> <br/> | ||
| + | |||
| + | RF signal from a single voxel has the form <br/> | ||
| + | <math>\begin{align} | ||
| + | r(t) &= f(x,y)e^{j\phi(t)} \\ | ||
| + | &= f(x,y)e^{j(\omega_0t + xk_x(t) + yk_y(t))} \\ | ||
| + | &= f(x,y)e^{j\omega_0t}e^{j(xk_x(t) + yk_y(t))} | ||
| + | \end{align}</math> | ||
| + | |||
| + | |||
| + | ---- | ||
| + | |||
| + | ==Received Signal from Selected Slice == | ||
| + | |||
| + | RF signal from the complete slice is given by <br/> | ||
| + | <math>\begin{align} | ||
| + | R(t) &= \int_{\mathbf{R}}\int_{\mathbf{R}}r(x,y,t)dxdy \\ | ||
| + | &= \int_{\mathbf{R}}\int_{\mathbf{R}} f(x,y)e^{j\omega_0t}e^{j(xk_x(t) + yk_y(t))}dxdy \\ | ||
| + | &= e^{j\omega_0t}\int_{\mathbf{R}}\int_{\mathbf{R}} f(x,y)e^{j(xk_x(t) + yk_y(t))}dxdy \\ | ||
| + | &= e^{j\omega_0t}F(-k_x(t),-k_y(t)) | ||
| + | \end{align}</math> | ||
| + | |||
| + | where <math>F(u,v) </math> is the CSFT of <math>f(x,y)</math> | ||
| + | |||
| + | |||
| + | ---- | ||
| + | |||
| + | ==K-Space Interpretation of Demodulated Signal== | ||
| + | |||
| + | RF signal from the complete slice is given by <br/> | ||
| + | <math>F(-k_x(t),-k_y(t))=R(t)e^{j\omega_0t}</math> | ||
| + | |||
| + | where<br/> | ||
| + | <math>k_x(t) = \int_0^t LG_x(\tau)d\tau</math> <br/> | ||
| + | <math>k_y(t) = \int_0^t LG_y(\tau)d\tau</math> | ||
| + | |||
| + | Strategy | ||
| + | *Scan partial frequencies by varying <math>k_x(t)</math> and <math>k_y(t)</math> <br/> | ||
| + | *Reconstruct image by performing the (inverse) CSFT | ||
| + | *<math>G_x(t)</math> and <math>G_y(t)</math> control velocity through K-space | ||
| + | |||
| + | |||
| + | ---- | ||
| + | |||
| + | ==Controlling K-Space Trajectory== | ||
| + | |||
| + | Relationships between gradient coil voltage and K-space <br/> | ||
| + | <math>\begin{align} | ||
| + | L_x\frac{di(t)}{dt} &= v_x(t) \quad G_x(t) = M_xi(t) \\ | ||
| + | L_y\frac{di(t)}{dt} &= v_y(t) \quad G_y(t) = M_yi(t) | ||
| + | \end{align}</math> | ||
| + | |||
| + | using this result in <br/> | ||
| + | <math>\begin{align} | ||
| + | k_x(t) &= \frac{LM_x}{L_x}\int_0^t\int_0^{\tau}v_x(s)dsd\tau \\ | ||
| + | k_y(t) &= \frac{LM_y}{L_y}\int_0^t\int_0^{\tau}v_y(s)dsd\tau | ||
| + | \end{align}</math> | ||
| + | |||
| + | <math>v_x(t)</math> and <math>v_y(t)</math> are like the accelerator peddles for <math>k_x(t)</math> and <math>k_y(t)</math>. | ||
| + | |||
| + | |||
| + | ---- | ||
| + | |||
| + | ==Echo Planar Imaging (EPI) Scan Pattern== | ||
| + | |||
| + | |||
| + | [[Image:intro_fig4.jpeg|400px|thumb|left|Fig 7: A commonly used raster scan pattern through K-space]] | ||
| + | |||
| + | <math>\begin{align} | ||
| + | k_x(t) &= L\int_0^t G_x(\tau)d\tau = \frac{LM_x}{L_x}\int_0^t\int_0^{\tau} v_x(s)dsd\tau \\ | ||
| + | k_y(t) &= L\int_0^t G_y(\tau)d\tau = \frac{LM_y}{L_y}\int_0^t\int_0^{\tau} v_y(s)dsd\tau | ||
| + | \end{align}</math> | ||
| + | |||
| + | |||
| + | ---- | ||
| + | |||
| + | ==Gradient Waveforms for EPI</math> | ||
| + | |||
| + | [[Image:intro_fig4.jpeg|400px|thumb|left|Fig 8: Gradient waveforms in <math>x</math> and <math>y</math>]] | ||
| + | |||
| + | [[Image:intro_fig4.jpeg|400px|thumb|left|Fig 9: Voltage waveforms in <math>x</math> and <math>y</math>]] | ||
| + | |||
| + | |||
Revision as of 08:20, 14 June 2013
- ↳ Topic 3: Magnetic Resonance Imaging
The Bouman Lectures on Image Processing
A sLecture by Maliha Hossain
Topic 3: Magnetic Resonance Imaging
© 2013
Contents
- 1 Excerpt from Prof. Bouman's Lecture
- 2 Accompanying Lecture Notes
- 2.1 MRI Attributes
- 2.2 Magnetic Resonance
- 2.3 The MRI Magnet
- 2.4 Magnetic Field Gradients
- 2.5 MRI Slice Select
- 2.6 Slice Select Pulse Design
- 2.7 Imaging the Selected Slice
- 2.8 Signal from a Single Voxel
- 2.9 Analysis of Phase
- 2.10 Received Signal from Selected Slice
- 2.11 K-Space Interpretation of Demodulated Signal
- 2.12 Controlling K-Space Trajectory
- 2.13 Echo Planar Imaging (EPI) Scan Pattern
- 2.14 References
- 2.15 Questions and comments
Excerpt from Prof. Bouman's Lecture
Accompanying Lecture Notes
Magnetic Resonance Imaging (MRI)
Definition
- Can be very high resolution
- No exposure to ionizing radiation
- Very flexible and programmable
- Tends to be expensive, noisy, and slow
MRI Attributes
- Based on magnetic resonance effect in atomic species
- Does not requires any ionizing radiation
- Numerous modalitites
- Conventional anatomical scans
- Functional MRI(fMRI)
- MRI Tagging
- Image formation
- RF excitation of magnetic resonance modes
- Magnetic field gradients modulate resonance frequency
- Reconstruction computed with inverse Fourier transform
- Fully programmable
- Requires an enormous (and very expensive) superconducting magnet
Magnetic Resonance
- Atom will precess at the Lamor frequency
$ \omega_0 = LM \ $
where
$ M $ is the magnitude of the ambient magnetic field
$ \omega_0 $ is the frequency of precession in radians per second
$ L $ is the Lamor constant and its value depends on the choice of atom
The MRI Magnet
- Large superconducting magnet
- Uniform field within bore
- Very large static magnetic field $ M_0 $
Magnetic Field Gradients
- Magnetic field magnitude at the location $ (x,y,z) $ has the form
$ M(x,y,z) = M_0 + xG_x + yG_y + zG_z \ $
- $ G_x $, $ G_y $ and $ G_z $ control magnetic field gradients
- Gradients can be changed with time
- Gradients are small compared to $ M_0 $
- For the time varying gradients,
$ M(x,y,z,t) = M_0 + xG_x(t) + yG_y(t) + zG_z(t) \ $
MRI Slice Select
Design RF pulse to excite protons in single slice
- Turn off $ x $ and $ y $ gradients
- Set $ z $ gradient to fix positive value, $ G_z > 0 $
- Use the fact that resonance frequency is given by
$ \omega = L(M_0+zG_z) $
Slice Select Pulse Design
- Design parameters
- Slice center$ = z_c $
- Slice thickness $ = \Delta z $
- Slice centered at $ z_c $ ⇒ pulse frequency
$ f_c = \frac{LM_0}{2\pi}+\frac{z_cLG_z}{2\pi} = f_0 + \frac{z_cLG_z}{2\pi} $
- Slice thickness $ \Delta z $ ⇒ pulse bandwidth
$ \Delta f = \frac{\Delta z LG_z}{2\pi} $
- Using the parameters. the pulse is given by
$ s(t) = e^{j2\pi f_ct}sinc(t\Delta f) $
and its CTFT is given by
$ S(f) = rect(\frac{f-f_c}{\Delta f}) $
Imaging the Selected Slice
- Precessing atoms radiate electromagnetic energy at RF frequencies
- Strategy
- Vary magnetic gradients along $ x $ and $ y $ axes
- Measure received RF signal
- Reconstruct image from RF measurements
Signal from a Single Voxel
RF signal from a single voxel has the form
$ r(x,y,t) = f(x,y)e^{j\phi(t)} \ $
$ f(x,y) $ voxel dependent weight
- Depends on properties of material in voxel
- Quantity of interest
- Typically "weighted" by T1, T2, or T3*
$ \phi(t) $ phase of received signal
- Can be modulated using $ G_x $ and $ G_y $ magnetic field gradients
- We assume that $ \phi(0) = 0 $
Analysis of Phase
Frequency = time derivative of phase
$ \begin{align} \frac{d\phi(t)}{dt} &= LM(x,y,t) \\ \phi(t) &= \int_0^t LM(x,y,\tau)d\tau \\ &= \int_0^t LM_0 + xLG_x(\tau) + yLG_y(\tau)d\tau \\ &= \omega_0t + xk_x(t) +yky(t) \end{align} $
where we define
$ \omega_0 = LM_0 $
$ k_x(t) = \int_0^t LG_x(\tau)d\tau $
$ k_y(t) = \int_0^t LG_y(\tau)d\tau $
RF signal from a single voxel has the form
$ \begin{align} r(t) &= f(x,y)e^{j\phi(t)} \\ &= f(x,y)e^{j(\omega_0t + xk_x(t) + yk_y(t))} \\ &= f(x,y)e^{j\omega_0t}e^{j(xk_x(t) + yk_y(t))} \end{align} $
Received Signal from Selected Slice
RF signal from the complete slice is given by
$ \begin{align} R(t) &= \int_{\mathbf{R}}\int_{\mathbf{R}}r(x,y,t)dxdy \\ &= \int_{\mathbf{R}}\int_{\mathbf{R}} f(x,y)e^{j\omega_0t}e^{j(xk_x(t) + yk_y(t))}dxdy \\ &= e^{j\omega_0t}\int_{\mathbf{R}}\int_{\mathbf{R}} f(x,y)e^{j(xk_x(t) + yk_y(t))}dxdy \\ &= e^{j\omega_0t}F(-k_x(t),-k_y(t)) \end{align} $
where $ F(u,v) $ is the CSFT of $ f(x,y) $
K-Space Interpretation of Demodulated Signal
RF signal from the complete slice is given by
$ F(-k_x(t),-k_y(t))=R(t)e^{j\omega_0t} $
where
$ k_x(t) = \int_0^t LG_x(\tau)d\tau $
$ k_y(t) = \int_0^t LG_y(\tau)d\tau $
Strategy
- Scan partial frequencies by varying $ k_x(t) $ and $ k_y(t) $
- Reconstruct image by performing the (inverse) CSFT
- $ G_x(t) $ and $ G_y(t) $ control velocity through K-space
Controlling K-Space Trajectory
Relationships between gradient coil voltage and K-space
$ \begin{align} L_x\frac{di(t)}{dt} &= v_x(t) \quad G_x(t) = M_xi(t) \\ L_y\frac{di(t)}{dt} &= v_y(t) \quad G_y(t) = M_yi(t) \end{align} $
using this result in
$ \begin{align} k_x(t) &= \frac{LM_x}{L_x}\int_0^t\int_0^{\tau}v_x(s)dsd\tau \\ k_y(t) &= \frac{LM_y}{L_y}\int_0^t\int_0^{\tau}v_y(s)dsd\tau \end{align} $
$ v_x(t) $ and $ v_y(t) $ are like the accelerator peddles for $ k_x(t) $ and $ k_y(t) $.
Echo Planar Imaging (EPI) Scan Pattern
$ \begin{align} k_x(t) &= L\int_0^t G_x(\tau)d\tau = \frac{LM_x}{L_x}\int_0^t\int_0^{\tau} v_x(s)dsd\tau \\ k_y(t) &= L\int_0^t G_y(\tau)d\tau = \frac{LM_y}{L_y}\int_0^t\int_0^{\tau} v_y(s)dsd\tau \end{align} $
==Gradient Waveforms for EPI</math>
References
- C. A. Bouman. ECE 637. Class Lecture. Digital Image Processing I. Faculty of Electrical Engineering, Purdue University. Spring 2013.
- G. Francis. Psy 200. "Introduction to Cognitive Psychology". Class Lecture Notes. Faculty of Psychological Sciences, Purdue University. Spring 2013.
Questions and comments
If you have any questions, comments, etc. please post them on this page
Back to the "Bouman Lectures on Image Processing" by Maliha Hossain