(New page: Category:2010 Fall ECE 438 Boutin ---- == Solution to HW7 == ---- Q1. <br/> Recall, the Discrete Fourier Transform is defined as follows - Definition: let x[n] be a DT signal with ...) |
|||
| (16 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
| − | + | <br> | |
---- | ---- | ||
| − | == Solution to HW7 == | + | |
| + | == Solution to [[Hw7ECE438F10|HW7]] == | ||
| + | |||
---- | ---- | ||
| − | Q1. <br | + | Q1. <br> |
| − | Recall, the Discrete Fourier Transform is defined as follows - | + | Recall, the Discrete Fourier Transform is defined as follows - |
| − | Definition: let x[n] be a DT signal with Period N. Then, | + | Definition: let x[n] be a DT signal with Period N. Then, |
| − | + | ||
| − | + | ||
| − | <math> x [n] = (1/N) \sum_{k=0}^{N-1} X[k].e^{j2\pi kn/N}</math> | + | <math> X [k] = \sum_{k=0}^{N-1} x[n].e^{-j2\pi kn/N}</math> |
| + | |||
| + | <math> x [n] = (1/N) \sum_{k=0}^{N-1} X[k].e^{j2\pi kn/N}</math> | ||
| + | |||
| + | <br> What is the relation between the DFT and the Fourier series coefficients of continuous periodic function x[n]? <br> The DFT of a sampled signal x[n] of length N is directly proportional to the Fourier series coefficients of the continuous periodic version of x[n]. <br> The DFT of the N samples comprising one period of x[n] equals N times the Fourier series coefficients. | ||
| + | |||
| + | Alternatively - <br> The fourier series coefficients of a periodic, bandlimited signal x are given by the DFT of one period of the samples of x, divided by N, where N is the DFT length and N is also the number of samples in each period of x. | ||
| + | |||
| + | Credit: Julius Smith III, stanford.edu | ||
---- | ---- | ||
| + | |||
<math>x_1[n]= e^{j \frac{2}{3} \pi n};</math> | <math>x_1[n]= e^{j \frac{2}{3} \pi n};</math> | ||
| − | Function's period N = 3, <br | + | Function's period N = 3, <br> Using IDFT, <br> <math>\begin{align} |
| − | Using IDFT, <br | + | x_1[n] &= \frac{1}{3} \sum_{k=0}^{2} X[k].e^{j2\pi kn/3} \\ |
| − | <math> | + | |
| − | \begin{align} | + | |
| − | + | ||
e^{j \frac{2}{3} \pi n} &= \frac{1}{3} \sum_{k=0}^{2} X[k].e^{j2\pi kn/3} \\ | e^{j \frac{2}{3} \pi n} &= \frac{1}{3} \sum_{k=0}^{2} X[k].e^{j2\pi kn/3} \\ | ||
| − | &= \frac{1}{3} \left[ X[0].e^{j0} + X[1].e^{j2\pi n/3} + X[2].e^{j2\pi (2/3)} \right] \\ | + | &= \frac{1}{3} \left[ X[0].e^{j0} + X[1].e^{j2\pi n/3} + X[2].e^{j2\pi n(2/3)} \right] \\ |
| − | &= \frac{1}{3} \left[ X[0] + X[1].e^{j2\pi n/3} + X[2].e^{j4\pi /3} \right] | + | &= \frac{1}{3} \left[ X[0] + X[1].e^{j2\pi n/3} + X[2].e^{j4\pi n/3} \right] |
| − | \end{align} | + | \end{align}</math> |
| − | </math> | + | |
| − | For the two sides to be equal, <br | + | For the two sides to be equal, <br> X[0] = 0 <br> X[1] = 3 <br> X[2] = 0 <br> |
| − | X[0] = 0 <br | + | |
| − | X[1] = 3 <br | + | |
| − | X[2] = 0 <br | + | |
| − | Plugging in we can verify, <br | + | Plugging in we can verify, <br> <math>\begin{align} |
| − | <math> | + | |
| − | \begin{align} | + | |
e^{j \frac{2}{3} \pi n} &= \frac{1}{3} \left[ 0 + 3.e^{j2\pi n/3} + 0 \right]\\ | e^{j \frac{2}{3} \pi n} &= \frac{1}{3} \left[ 0 + 3.e^{j2\pi n/3} + 0 \right]\\ | ||
e^{j \frac{2}{3} \pi n} &= \frac{1}{3} 3.e^{j2\pi n/3} \\ | e^{j \frac{2}{3} \pi n} &= \frac{1}{3} 3.e^{j2\pi n/3} \\ | ||
e^{j \frac{2}{3} \pi n} &= e^{j \frac{2}{3} \pi n} | e^{j \frac{2}{3} \pi n} &= e^{j \frac{2}{3} \pi n} | ||
| − | \end{align} | + | \end{align}</math> |
| − | </math> | + | |
| − | So our three selected values for X[k] are correct. Thus <br | + | So our three selected values for X[k] are correct. Thus <br> <math>X[k] = \begin{cases} |
| − | <math> | + | |
| − | X[k] = \begin{cases} | + | |
3, & k = 1 \\ | 3, & k = 1 \\ | ||
0, & \mbox{else} | 0, & \mbox{else} | ||
| − | \end{cases} | + | \end{cases}</math> |
| − | </math> | + | |
| + | ---- | ||
| − | + | <math>x_2[n]= e^{j \frac{2}{\sqrt{3}} \pi n};</math> | |
| − | < | + | Function x2[n] is aperiodic. Let's see why - <br> Assume x2[n] is periodic, then <br> <math>e^{j \frac{2}{\sqrt{3}} \pi n} = e^{j \frac{2}{\sqrt{3}} \pi (n + N)}</math> for function to be periodic, where N is an integer <br> <math>e^{j \frac{2}{\sqrt{3}} \pi n} = e^{j \frac{2}{\sqrt{3}} \pi n}e^{j \frac{2}{\sqrt{3}} \pi N}</math> <br> <math>e^{j \frac{2}{\sqrt{3}} \pi n} = e^{j \frac{2}{\sqrt{3}} \pi n}.(1)</math> <br> <math>e^{j \frac{2}{\sqrt{3}} \pi N} = 1</math> <br> For this to be true - <br> <math>j \frac{2}{\sqrt{3}} \pi N = j 2\pi n,</math> where n is an integer<br> <math>N = n\sqrt{3}</math> <br> N is not an integer and this contradicts our assumption, proving that it cannot be true.<br> Thus, x_2[n] is aperiodic and we cannot apply the DFT. <br> |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
---- | ---- | ||
<math>x_3[n]= e^{j \frac{4}{3} \pi n};</math> | <math>x_3[n]= e^{j \frac{4}{3} \pi n};</math> | ||
| − | Function's period N = 3, <br | + | Function's period N = 3, <br> Using IDFT, <br> <math>\begin{align} |
| − | Using IDFT, <br | + | x_3[n] &= \frac{1}{3} \sum_{k=0}^{2} X[k].e^{j2\pi kn/3} \\ |
| − | <math> | + | |
| − | \begin{align} | + | |
| − | + | ||
e^{j \frac{4}{3} \pi n} &= \frac{1}{3} \sum_{k=0}^{2} X[k].e^{j2\pi kn/3} \\ | e^{j \frac{4}{3} \pi n} &= \frac{1}{3} \sum_{k=0}^{2} X[k].e^{j2\pi kn/3} \\ | ||
&= \frac{1}{3} \left[ X[0].e^{j0} + X[1].e^{j2\pi n/3} + X[2].e^{j2\pi n(2/3)} \right] \\ | &= \frac{1}{3} \left[ X[0].e^{j0} + X[1].e^{j2\pi n/3} + X[2].e^{j2\pi n(2/3)} \right] \\ | ||
&= \frac{1}{3} \left[ X[0] + X[1].e^{j2\pi n/3} + X[2].e^{j4\pi n/3} \right] | &= \frac{1}{3} \left[ X[0] + X[1].e^{j2\pi n/3} + X[2].e^{j4\pi n/3} \right] | ||
| − | \end{align} | + | \end{align}</math> |
| − | </math> | + | |
| − | For the two sides to be equal, <br | + | For the two sides to be equal, <br> X[0] = 0 <br> X[1] = 0 <br> X[2] = 3 <br> |
| − | X[0] = 0 <br | + | |
| − | X[1] = 0 <br | + | |
| − | X[2] = 3 <br | + | |
| − | <math> | + | <math>X[k] = \begin{cases} |
| − | X[k] = \begin{cases} | + | |
3, & k = 2 \\ | 3, & k = 2 \\ | ||
0, & \mbox{else} | 0, & \mbox{else} | ||
| − | \end{cases} | + | \end{cases}</math> |
| − | </math> | + | |
---- | ---- | ||
| − | <math>x_4[n]= e^{j \frac{2}{1000} \pi n};</math> | + | |
| − | Function's period N = 1000, <br | + | <math>x_4[n]= e^{j \frac{2}{1000} \pi n};</math> Function's period N = 1000, <br> Using IDFT, <br> <math>\begin{align} |
| − | Using IDFT, <br | + | x_4[n] &= \frac{1}{1000} \sum_{k=0}^{999} X[k].e^{j2\pi kn/1000} \\ |
| − | <math> | + | |
| − | \begin{align} | + | |
| − | + | ||
e^{j \frac{2}{1000} \pi n} &= \frac{1}{1000} \sum_{k=0}^{999} X[k].e^{j2\pi kn/1000} \\ | e^{j \frac{2}{1000} \pi n} &= \frac{1}{1000} \sum_{k=0}^{999} X[k].e^{j2\pi kn/1000} \\ | ||
&= \frac{1}{1000} \left[ X[0].e^{j0} + X[1].e^{j2\pi n/1000} + X[2].e^{j2\pi n(2/1000)} + ... \right] \\ | &= \frac{1}{1000} \left[ X[0].e^{j0} + X[1].e^{j2\pi n/1000} + X[2].e^{j2\pi n(2/1000)} + ... \right] \\ | ||
&= \frac{1}{1000} \left[ X[0] + X[1].e^{j2\pi n/1000} + X[2].e^{j4\pi n/1000} + ... \right] | &= \frac{1}{1000} \left[ X[0] + X[1].e^{j2\pi n/1000} + X[2].e^{j4\pi n/1000} + ... \right] | ||
| − | \end{align} | + | \end{align}</math> |
| − | </math> | + | |
| − | For the two sides to be equal, <br | + | For the two sides to be equal, <br> X[0] = 0 <br> X[1] = 1000 <br> X[2] = 0 <br> |
| − | X[0] = 0 <br | + | |
| − | X[1] = 1000 <br | + | |
| − | X[2] = 0 <br | + | |
| − | <math> | + | <math>X[k] = \begin{cases} |
| − | X[k] = \begin{cases} | + | |
1000, & k = 1 \\ | 1000, & k = 1 \\ | ||
0, & \mbox{else} | 0, & \mbox{else} | ||
| − | \end{cases} | + | \end{cases}</math> |
| − | </math> | + | |
---- | ---- | ||
| − | <math>x_5[n]= e^{-j \frac{2}{1000} \pi n};</math> | + | <math>x_5[n]= e^{-j \frac{2}{1000} \pi n};</math> Function's period N = 1000, <br> <math>\begin{align} |
| − | Function's period N = 1000, <br | + | |
| − | <math> | + | |
| − | \begin{align} | + | |
x_5[n]&= e^{-j \frac{2}{1000} \pi n}.1 \\ | x_5[n]&= e^{-j \frac{2}{1000} \pi n}.1 \\ | ||
&= e^{-j \frac{2}{1000} \pi n}.e^{-j 2\pi n} \\ | &= e^{-j \frac{2}{1000} \pi n}.e^{-j 2\pi n} \\ | ||
&= e^{j 2\pi n(1 - (1/1000))} \\ | &= e^{j 2\pi n(1 - (1/1000))} \\ | ||
&= e^{j 2\pi n\frac{999}{1000} } \\ | &= e^{j 2\pi n\frac{999}{1000} } \\ | ||
| − | \end{align} | + | \end{align}</math> |
| − | </math> | + | |
| − | Using IDFT, <br | + | Using IDFT, <br> <math>\begin{align} |
| − | <math> | + | x_5[n] &= \frac{1}{1000} \sum_{k=0}^{999} X[k].e^{j2\pi kn/1000} \\ |
| − | \begin{align} | + | |
| − | + | ||
e^{j 2\pi n\frac{999}{1000}} &= \frac{1}{1000} \sum_{k=0}^{999} X[k].e^{j2\pi kn/1000} \\ | e^{j 2\pi n\frac{999}{1000}} &= \frac{1}{1000} \sum_{k=0}^{999} X[k].e^{j2\pi kn/1000} \\ | ||
| − | &= \frac{1}{1000} \left[ X[0].e^{j0} + X[1].e^{j2\pi n/1000} + X[2].e^{j2\pi n(2/1000)}+ ... + X[999].e^{j2\pi (999/1000)} \right] \\ | + | &= \frac{1}{1000} \left[ X[0].e^{j0} + X[1].e^{j2\pi n/1000} + X[2].e^{j2\pi n(2/1000)}+ ... + X[999].e^{j2\pi n(999/1000)} \right] \\ |
| − | &= \frac{1}{1000} \left[ X[0] + X[1].e^{j2\pi n/1000} + X[2].e^{j2\pi n (2/1000)} + ... + X[999].e^{j2\pi (999/1000)} \right] \\ | + | &= \frac{1}{1000} \left[ X[0] + X[1].e^{j2\pi n/1000} + X[2].e^{j2\pi n (2/1000)} + ... + X[999].e^{j2\pi n(999/1000)} \right] \\ |
| − | \end{align} | + | \end{align}</math> |
| − | </math> | + | |
| − | For the two sides to be equal, <br | + | For the two sides to be equal, <br> X[0] = 0 <br> X[1] = 0 <br> X[2] = 0 <br> X[999] = 1000 <br> |
| − | X[0] = 0 <br | + | |
| − | X[1] = 0 <br | + | |
| − | X[2] = 0 <br | + | |
| − | X[999] = 1000 <br | + | |
| − | <math> | + | <math>X[k] = \begin{cases} |
| − | X[k] = \begin{cases} | + | |
1000, & k = 999 \\ | 1000, & k = 999 \\ | ||
0, & \mbox{else} | 0, & \mbox{else} | ||
| − | \end{cases} | + | \end{cases}</math> |
| + | |||
| + | ---- | ||
| + | |||
| + | <math>\begin{align} | ||
| + | x_6[n] &= \cos\left( \frac{2}{1000} \pi n\right) \\ | ||
| + | &= \frac{1}{2}\left( e^{j\frac{2\pi n}{1000}} + e^{-j\frac{2\pi n}{1000}} \right) \\ | ||
| + | &= \frac{1}{2} (x_4[n] + x_5[n]) \\ | ||
| + | \end{align}</math> | ||
| + | |||
| + | We have an additional (1/2) to factor into the final coefficients, giving us - <br> <math>X[k] = \begin{cases} | ||
| + | 500, & k = 1 \\ | ||
| + | 500, & k = 999 \\ | ||
| + | 0, & \mbox{else} | ||
| + | \end{cases}</math> | ||
| + | |||
| + | ---- | ||
| + | |||
| + | <math>\begin{align} | ||
| + | x_7[n] &= \cos^2\left( \frac{2}{1000} \pi n\right) \\ | ||
| + | &= \left[ \frac{1}{2}\left( e^{j\frac{2\pi n}{1000}} + e^{-j\frac{2\pi n}{1000}} \right)\right]^2 \\ | ||
| + | &= \frac{1}{4}\left( e^{j\frac{4\pi n}{1000}} + 2 + e^{-j\frac{4\pi n}{1000}} \right) \\ | ||
| + | &= \frac{1}{4}\left( 2 + e^{j2\pi n\frac{2}{1000}} + e^{-j2\pi n\frac{2}{1000}}e^{-j2\pi n} \right) \\ | ||
| + | &= \frac{1}{4}\left( 2 + e^{j2\pi n\frac{2}{1000}} + e^{j2\pi n\frac{998}{1000}} \right) \\ | ||
| + | \end{align}</math> | ||
| + | |||
| + | Function's period N = 1000, <br> Using IDFT, <br> <math>\begin{align} | ||
| + | x_7[n] &= \frac{1}{1000} \sum_{k=0}^{999} X[k].e^{j2\pi kn/1000} \\ | ||
| + | &= \frac{1}{1000} \left[ X[0] + X[1].e^{j2\pi n/1000} + X[2].e^{j2\pi n(2/1000)} + ... X[998].e^{j2\pi n(998/1000)} + X[999].e^{j2\pi n(999/1000)} \right] \\ | ||
| + | \end{align}</math> | ||
| + | |||
| + | Comparing LHS and RHS, <br> X[0] = 500 <br> X[1] = 0 <br> X[2] = 250 <br> ... <br> X[998] = 250 <br> X[999] = 0 <br> | ||
| + | |||
| + | <math>X[k] = \begin{cases} | ||
| + | 500, & k = 0 \\ | ||
| + | 250, & k = 2 \\ | ||
| + | 250, & k = 998 \\ | ||
| + | 0, & \mbox{else} | ||
| + | \end{cases}</math> | ||
| + | |||
| + | ---- | ||
| + | |||
| + | <math>\begin{align} | ||
| + | x_8[n]= (-j)^n \\ | ||
| + | &= (e^{-j \pi /2})^n \\ | ||
| + | &= e^{-j \pi n/2} \\ | ||
| + | &= e^{-j \pi n/2}e^{j 2\pi} \\ | ||
| + | &= e^{j 2\pi (3n/4)} \\ | ||
| + | |||
| + | \end{align}</math> | ||
| + | |||
| + | Function's period N = 4, <br> Using IDFT, <br> <math>\begin{align} | ||
| + | x_8[n] &= \frac{1}{4} \sum_{k=0}^{3} X[k].e^{j2\pi kn/4} \\ | ||
| + | e^{j 2\pi (3n/4)} &= \frac{1}{4} \sum_{k=0}^{3} X[k].e^{j2\pi kn/4} \\ | ||
| + | &= \frac{1}{4} \left[ X[0].e^{j0} + X[1].e^{j2\pi n/4} + X[2].e^{j2\pi n(2/4)} + X[3].e^{j2\pi n(3/4)}\right] \\ | ||
| + | &= \frac{1}{4} \left[ X[0] + X[1].e^{j\pi n/2} + X[2].e^{j\pi n} + X[3].e^{j\pi n(3/2)} \right] | ||
| + | \end{align}</math> | ||
| + | |||
| + | For the two sides to be equal, <br> X[0] = 0 <br> X[1] = 0 <br> X[2] = 0 <br> X[3] = 4 <br> | ||
| + | |||
| + | <math>X[k] = \begin{cases} | ||
| + | 4, & k = 3 \\ | ||
| + | 0, & \mbox{else} | ||
| + | \end{cases}</math> | ||
| + | |||
| + | ---- | ||
| + | |||
| + | Q2. | ||
| + | |||
| + | <math>y_1[n]= \frac{x[n]+x[n-1]}{2} </math><br> | ||
| + | |||
| + | Applying Z-transform on both sides and grouping terms, we can obtain the transfer function<br/> | ||
| + | |||
| + | <math>\begin{align} | ||
| + | Y_1[z]&= \frac{X[z]+X[z].z^{-1}}{2} \\ | ||
| + | \frac{Y_1[z]}{X[z]}&= \frac{1+z^{-1}}{2} \\ | ||
| + | H_1[z] &= \frac{1+z^{-1}}{2} \\ | ||
| + | \end{align}</math> | ||
| + | |||
| + | Frequency Response H_1(<span class="texhtml">ω</span>),<br> <math>\begin{align} | ||
| + | H_1[e^{j\omega }] &= \frac{1+e^{-j\omega }}{2} \\ | ||
| + | &= e^{-j\frac{\omega }{2}} \left( \frac{e^{j\frac{\omega }{2}}+e^{-j\frac{\omega }{2}}}{2} \right) \\ | ||
| + | &= e^{-j\frac{\omega }{2}} cos \left( \frac{\omega }{2} \right) \\ | ||
| + | \end{align}</math> | ||
| + | |||
| + | ---- | ||
| + | |||
| + | <math>y_2[n]= \frac{x[n]-x[n-1]}{2}</math> | ||
| + | |||
| + | Applying Z-transform on both sides and grouping terms, we can obtain the transfer function<br> | ||
| + | |||
| + | <math>\begin{align} | ||
| + | Y_2[z]&= \frac{X[z]-X[z].z^{-1}}{2} \\ | ||
| + | \frac{Y_2[z]}{X[z]}&= \frac{1-z^{-1}}{2} \\ | ||
| + | H_2[z] &= \frac{1-z^{-1}}{2} \\ | ||
| + | \end{align}</math> | ||
| + | |||
| + | Frequency Response H_2(<span class="texhtml">ω</span>),<br> <math>\begin{align} | ||
| + | H_2[e^{j\omega }] &= \frac{1-e^{-j\omega }}{2} \\ | ||
| + | &= e^{-j\frac{\omega }{2}} \left( \frac{e^{j\frac{\omega }{2}}-e^{-j\frac{\omega }{2}}}{2} \right) \\ | ||
| + | &= je^{-j\frac{\omega }{2}} \left( \frac{e^{j\frac{\omega }{2}}-e^{-j\frac{\omega }{2}}}{2j} \right) \\ | ||
| + | &= je^{-j\frac{\omega }{2}} sin \left( \frac{\omega }{2} \right) \\ | ||
| + | \end{align}</math> | ||
| + | |||
| + | ---- | ||
| + | |||
| + | <math>y_3[n]= \frac{x[n+1]+x[n]+x[n-1]}{3} </math><br/> | ||
| + | Applying Z-transform on both sides and grouping terms, we can obtain the transfer function<br/> | ||
| + | |||
| + | <math>\begin{align} | ||
| + | Y_3[z]&= \frac{X[z].z+ X[z] + X[z].z^{-1}}{3} \\ | ||
| + | \frac{Y_3[z]}{X[z]}&= \frac{z(1+z^{-1} + z^{-2})}{3} \\ | ||
| + | H_3[z] &= \frac{1+z^{-1} + z^{-2}}{3z^{-1}} \\ | ||
| + | \end{align}</math> | ||
| + | |||
| + | Frequency Response H_3(<span class="texhtml">ω</span>),<br> <math>\begin{align} | ||
| + | H_3[e^{j\omega }] &= \frac{1+e^{-j\omega }+e^{-j2\omega }}{3e^{-j\omega }} \\ | ||
| + | &= \frac{e^{-j\omega }+e^{-j\omega }(e^{j\omega } + e^{-j\omega })}{3e^{-j\omega }} \\ | ||
| + | &= \frac{e^{-j\omega }(1+2cos(\omega ))}{3e^{-j\omega }} \\ | ||
| + | &= \frac{1+2cos(\omega )}{3} \\ | ||
| + | \end{align}</math> | ||
| + | |||
| + | ---- | ||
| + | |||
| + | <math>y_4[n]= \frac{x[n+1]-2 x[n]+x[n-1]}{4}. </math><br/> | ||
| + | Applying Z-transform on both sides and grouping terms, we can obtain the transfer function<br/> | ||
| + | |||
| + | <math>\begin{align} | ||
| + | Y_4[z]&= \frac{X[z].z- 2X[z] + X[z].z^{-1}}{4} \\ | ||
| + | \frac{Y_3[z]}{X[z]}&= \frac{z(1-2z^{-1} + z^{-2})}{4} \\ | ||
| + | H_3[z] &= \frac{1-2z^{-1} + z^{-2}}{4z^{-1}} \\ | ||
| + | \end{align}</math> | ||
| + | |||
| + | Frequency Response H_3(<span class="texhtml">ω</span>),<br> <math>\begin{align} | ||
| + | H_4[e^{j\omega }] &= \frac{1-2e^{-j\omega }+e^{-j2\omega }}{4e^{-j\omega }} \\ | ||
| + | &= \frac{-2e^{-j\omega }+ 2 e^{-j\omega }\frac{(e^{j\omega } + e^{-j\omega })}{2}}{4e^{-j\omega }} \\ | ||
| + | &= \frac{2e^{-j\omega }(cos(\omega )-1)}{4e^{-j\omega }} \\ | ||
| + | &= \frac{cos(\omega )-1}{2} \\ | ||
| + | \end{align}</math> | ||
| + | |||
| + | ---- | ||
| + | |||
| + | Q3. | ||
| + | |||
| + | a. Substituting values directly would yield the following - <br> | ||
| + | |||
| + | <math>\begin{align} | ||
| + | n &= ...\text{ -3, -2, -1, 0, 1, 2, 3, 4, 5} ...\\ | ||
| + | y\left[n\right] &= ...\text{ 0, -2, -4, -1, 2, -1, -4, -2, 0} ...\\ | ||
| + | \end{align}</math> | ||
| + | |||
| + | b. h[n] = ? <br> Substitute x[n] = <span class="texhtml">δ[''n'']</span> to obtain y[n] = h[n], <br> h[n] = <span class="texhtml">δ[''n'']</span> + 2<span class="texhtml">δ[''n'' − 1]</span> + <span class="texhtml">δ[''n'' − 2]</span> <br> | ||
| + | |||
| + | Now x[n] = -2<span class="texhtml">δ[''n'' + 2]</span> + <span class="texhtml">δ[''n'']</span> - 2<span class="texhtml">δ[''n'' − 2]</span> <br> | ||
| + | |||
| + | <math>\begin{align} | ||
| + | y[n] &= x[n] * h[n] \\ | ||
| + | &= (-2\delta[n+2] + \delta[n] - 2\delta[n-2]) * (\delta[n] + 2\delta[n-1] + \delta[n-2]) \\ | ||
| + | &= -2\delta[n+2] - 4\delta[n+1] -2\delta[n] + \delta[n] + 2\delta[n-1] + \delta[n-2] - 2\delta[n-2] - 4\delta[n-3] - 2\delta[n-4] \\ | ||
| + | &= -2\delta[n+2] - 4\delta[n+1] - \delta[n] + 2\delta[n-1] - \delta[n-2] - 4\delta[n-3] - 2\delta[n-4] \\ | ||
| + | \end{align}</math> | ||
| + | |||
| + | c. x[n] = <span class="texhtml">''e''<sup>''j''ω''n''</sup></span> <br> (i) <br> <math>\begin{align} | ||
| + | y[n] &= e^{j\omega n} + 2 e^{j\omega (n-1)} + e^{j\omega (n-2)} \\ | ||
| + | &= e^{j\omega n}(1 + 2 e^{-j\omega } + e^{-2j\omega }) \\ | ||
| + | H(e^{j\omega}) &= 1 + 2 e^{-j\omega } + e^{-2j\omega } \\ | ||
| + | \end{align}</math> | ||
| + | |||
| + | (ii) <br> h[n] = <span class="texhtml">δ[''n'']</span> + 2<span class="texhtml">δ[''n'' − 1]</span> + <span class="texhtml">δ[''n'' − 2]</span> <br> H(<span class="texhtml">''e''<sup>''j''ω</sup></span>) = 1 + 2 <span class="texhtml">''e''<sup> − ''j''ω</sup></span> + <span class="texhtml">''e''<sup> − 2''j''ω</sup></span> <br> | ||
| + | |||
| + | (i) and (ii) are the same.<br> | ||
| + | |||
| + | d. x[n] = -2<span class="texhtml">δ[''n'' + 2]</span> + <span class="texhtml">δ[''n'']</span> - 2<span class="texhtml">δ[''n'' − 2]</span> <br> X(<span class="texhtml">''e''<sup>''j''ω</sup></span>) = -2 <span class="texhtml">''e''<sup>2''j''ω</sup></span> + 1 - 2<span class="texhtml">''e''<sup> − 2''j''ω</sup></span> <br> | ||
| + | |||
| + | <math>\begin{align} | ||
| + | Y(e^{j\omega}) &= X(e^{j\omega})H(e^{j\omega}) \\ | ||
| + | &= (-2e^{2j\omega } + 1 - 2e^{-2j\omega } ).(1 + 2e^{-j\omega } + e^{-2j\omega }) \\ | ||
| + | &= -2e^{2j\omega } - 4e^{j\omega } - 2 + 1 + 2e^{-j\omega } + 2e^{-2j\omega } - 2e^{-2j\omega } - 4e^{-3j\omega } - 2e^{-4j\omega } \\ | ||
| + | &= -2e^{2j\omega } - 4e^{j\omega } - 1 - 2e^{-j\omega } - e^{-2j\omega } - 4e^{-3j\omega } - 2e^{-4j\omega } \\ | ||
| + | \\ | ||
| + | \text{Using Inverse DTFT,} \\ | ||
| + | y[n] &= -2\delta[n+2] - 4\delta[n+1] - \delta[n] + 2\delta[n-1] - \delta[n-2] - 4\delta[n-3] - 2\delta[n-4] \\ | ||
| + | \end{align}</math> | ||
| + | |||
| + | All 3 approaches lead to the same y[n]. | ||
| + | |||
| + | ---- | ||
| + | |||
| + | Q4. <math>H(z)= \frac{1-\frac{1}{2}z^{-2}} | ||
| + | {1-\frac{1}{\sqrt{2}} z^{-1} +\frac{1}{4} z^{-2}}</math> | ||
| + | |||
| + | :a. Sketch the locations of the poles and zeros. | ||
| + | |||
| + | <math>\begin{align} | ||
| + | H(z) &= \frac{1-\frac{1}{2}z^{-2}}{1-\frac{1}{\sqrt{2}} z^{-1} +\frac{1}{4} z^{-2}} \\ | ||
| + | H(z) &= \frac{(z+\frac{1}{\sqrt{2}})(z-\frac{1}{\sqrt{2}})} | ||
| + | { (z-(\frac{1}{2\sqrt{2}} + j\frac{1}{2\sqrt{2}}))(z-(\frac{1}{2\sqrt{2}} - j\frac{1}{2\sqrt{2}})) } \\ | ||
| + | \end{align}</math> | ||
| + | |||
| + | Zeros: <br> <math>z_1 = \frac{1}{\sqrt{2}}, z_2 = -\frac{1}{\sqrt{2}}</math><br> Poles: <br> <math>p_1 = \frac{1}{2\sqrt{2}} + j\frac{1}{2\sqrt{2}}, p_2 = \frac{1}{2\sqrt{2}} - j\frac{1}{2\sqrt{2}}</math> | ||
| + | |||
| + | [[Image:Zp1.jpg]] | ||
| + | |||
| + | :b. Determine the magnitude and phase of the frequency response <span class="texhtml">''H''(ω)</span>, for | ||
| + | |||
| + | <math>\omega = 0</math> <br/> | ||
| + | [[Image:Zp2.jpg]] | ||
| + | <math> | ||
| + | \left| H(e^{j\omega}) \right| = \left| H(e^{j0}) \right| = \left| H(z=1) \right|</math><br> <math> = \left| \frac{(1+\frac{1}{\sqrt{2}})(1-\frac{1}{\sqrt{2}})} { (1-(\frac{1}{2\sqrt{2}} + j\frac{1}{2\sqrt{2}}))(1-(\frac{1}{2\sqrt{2}} - j\frac{1}{2\sqrt{2}})) } \right| = 0.921</math><br> <math>\angle H(e^{j0}) = \angle c + \angle d - \angle a - \angle b = 0</math> | ||
| + | |||
| + | <br> <math>\omega =\frac{\pi}{4}</math><br> | ||
| + | [[Image:Zp3.JPG]] | ||
| + | <math> | ||
| + | \left| H(e^{j\omega}) \right| = \left| H(e^{j\frac{\pi}{4}}) \right| = \left| H(z=\frac{1}{\sqrt{2}} + j\frac{1}{\sqrt{2}}) \right|</math><br> | ||
| + | <math> = \left| \frac{(\frac{1}{\sqrt{2}} + j\frac{1}{\sqrt{2}}+\frac{1}{\sqrt{2}})(\frac{1}{\sqrt{2}} + j\frac{1}{\sqrt{2}}-\frac{1}{\sqrt{2}})} { (\frac{1}{\sqrt{2}} + j\frac{1}{\sqrt{2}}-(\frac{1}{2\sqrt{2}} + j\frac{1}{2\sqrt{2}}))(\frac{1}{\sqrt{2}} + j\frac{1}{\sqrt{2}}-(\frac{1}{2\sqrt{2}} - j\frac{1}{2\sqrt{2}})) } \right| = 2 | ||
</math> | </math> | ||
| + | <br> | ||
| + | <math>\angle H(e^{j\frac{\pi}{4}}) = \angle c + \angle d - \angle a - \angle b = \frac{\pi}{2} + arctan^{-1} \left( \frac{\frac{1}{\sqrt{2}}}{\sqrt{2}} \right) - \frac{\pi}{4} - arctan^{-1} \left( \frac{\frac{1}{\sqrt{2}} + \frac{1}{2\sqrt{2}}}{1-\sqrt{2}-\frac{1}{2\sqrt{2}}} \right) = 0</math> | ||
| + | |||
| + | <math>\omega =\frac{\pi}{2}</math><br> [[Image:Zp4.jpg]] <math>\left| H(e^{j\omega}) \right| = \left| H(e^{j\frac{\pi}{2}}) \right| = \left| H(z=j) \right|</math><br> | ||
| + | |||
| + | <math> = \left| \frac{(j+\frac{1}{\sqrt{2}})(j-\frac{1}{\sqrt{2}})} { (j-(\frac{1}{2\sqrt{2}} + j\frac{1}{2\sqrt{2}}))(j-(\frac{1}{2\sqrt{2}} - j\frac{1}{2\sqrt{2}})) } \right| = 1.455</math><br> | ||
| + | |||
| + | <br> | ||
| + | <math>\angle H(e^{j\frac{\pi}{2}}) = (\angle c + \angle d) - \angle a - \angle b = (\pi) - (arctan^{-1}\left( \frac{1-\frac{1}{2\sqrt{2}}}{\frac{-1}{\sqrt{2}}} \right) + \pi) - (arctan^{-1}\left( \frac{1+\frac{1}{2\sqrt{2}}}{1-\frac{1}{\sqrt{2}}} \right) + \pi) = -0.7563 | ||
| + | </math> | ||
| + | |||
| + | <math>\omega =\frac{3\pi}{4}</math><br/> | ||
| + | [[Image:Zp5.JPG]] | ||
| + | <math>\left| H(e^{j\omega}) \right| = \left| H(e^{j\frac{3\pi}{4}}) \right| = \left| H(z=\frac{-1}{\sqrt{2}} + j\frac{1}{\sqrt{2}}) \right|</math><br> | ||
| + | |||
| + | <math> = \left| \frac{(\frac{-1}{\sqrt{2}} + j\frac{1}{\sqrt{2}}+\frac{1}{\sqrt{2}})(\frac{-1}{\sqrt{2}} + j\frac{1}{\sqrt{2}}-\frac{1}{\sqrt{2}})} { (\frac{-1}{\sqrt{2}} + j\frac{1}{\sqrt{2}}-(\frac{1}{2\sqrt{2}} + j\frac{1}{2\sqrt{2}}))(\frac{-1}{\sqrt{2}} + j\frac{1}{\sqrt{2}}-(\frac{1}{2\sqrt{2}} - j\frac{1}{2\sqrt{2}})) } \right| = \frac{2}{3} | ||
| + | </math> | ||
| + | |||
| + | <math>\angle H(e^{j\frac{3\pi}{4}}) = \angle c + \angle d - \angle a - \angle b = (arctan^{-1}\left( \frac{\frac{1}{\sqrt{2}}}{\frac{-2}{\sqrt{2}}} \right) + \pi) + \frac{\pi}{2} - (arctan^{-1}\left( \frac{\frac{1}{\sqrt{2}} - \frac{1}{2\sqrt{2}}}{\frac{1}{\sqrt{2}} - \frac{1}{2\sqrt{2}}} \right) + \pi) + \frac{3\pi}{4} = -0.9273 | ||
| + | </math> | ||
| + | |||
| + | |||
| + | <math>\omega =\pi</math><br/> | ||
| + | [[Image:Zp6.JPG]] | ||
| + | <math> | ||
| + | \left| H(e^{j\omega}) \right| = \left| H(e^{j\pi}) \right| = \left| H(z=-1) \right|</math><br> | ||
| + | <math> = \left| \frac{(-1+\frac{1}{\sqrt{2}})(-1-\frac{1}{\sqrt{2}})} { (-1-(\frac{1}{2\sqrt{2}} + j\frac{1}{2\sqrt{2}}))(-1-(\frac{1}{2\sqrt{2}} - j\frac{1}{2\sqrt{2}})) } \right| = 0.255 | ||
| + | </math><br> | ||
| + | |||
| + | <math>\angle H(e^{j\pi}) = (\angle c + \angle d) - \angle a - \angle b = 2\pi - 0 - 0 = 2\pi</math> | ||
| + | |||
| + | c. Is the system stable? Explain why or why not? <br/> | ||
| + | The system is causal and the ROC extends outwards from the outermost pole since |<math>p_1</math>| = |<math>p_2</math>| < 1 and this ROC contains the unit circle. Therefore the system is stable. <br/> | ||
| + | |||
| + | d. Find the difference equation for y[n] in terms of x[n], corresponding to this transfer function H(z). <br/> | ||
| + | |||
| + | <math>H(z) = \frac{Y(z)}{X(z)} = \frac{1-\frac{1}{2}z^{-2}}{1-\frac{1}{\sqrt{2}} z^{-1} +\frac{1}{4} z^{-2}}</math><br/> | ||
| + | <math>Y(z)(1-\frac{1}{\sqrt{2}} z^{-1} +\frac{1}{4} z^{-2}) = X(z)(1-\frac{1}{2}z^{-2})</math><br/> | ||
| + | |||
| + | Taking inverse,<br/> | ||
| + | <math>y[n]-\frac{1}{\sqrt{2}}y[n-1] + \frac{1}{4}y[n-2] = x[n] - \frac{1}{2}x[n-2]</math><br/> | ||
| + | <math>y[n] = x[n] - \frac{1}{2}x[n-2] +\frac{1}{\sqrt{2}}y[n-1] - \frac{1}{4}y[n-2]</math><br/> | ||
| + | <br> | ||
---- | ---- | ||
| − | <math> | + | Q5.<br/> |
| + | <math> | ||
| + | y[n]=\frac{1}{8} \left( x[n]+x[n-1]+x[n-2]+x[n-3]+x[n-4]+x[n-5]+x[n-6]+x[n-7]\right) | ||
| + | </math> | ||
| + | a.<br/> | ||
| + | <math> | ||
| + | h[n]=\frac{1}{8} \left( \delta[n]+\delta[n-1]+\delta[n-2]+\delta[n-3]+\delta[n-4]+\delta[n-5]+\delta[n-6]+\delta[n-7] \right) | ||
| + | </math> <br/> | ||
| + | This is a finite duration response.<br/> | ||
| + | b. | ||
| + | <math> | ||
| + | H[z]=\frac{1}{8} \left( 1+z^{-1}+z^{-2}+z^{-3}+z^{-4}+z^{-5}+z^{-6}+z^{-7} \right) | ||
| + | </math> <br/> | ||
| + | <math> | ||
| + | H[z]=\frac{1}{8} \left( \frac{1-z^{-8}}{1-z^{-1}} \right) | ||
| + | </math> <br/> | ||
| − | + | c. | |
| − | + | ||
| + | <math> | ||
| + | H[z]=\frac{1}{8} \left( \frac{z^{8}-1}{z^{7}(z-1)} \right) | ||
| + | </math> <br/> | ||
| + | [[Image:Zp7.jpg]] | ||
| + | <br/> | ||
| + | Poles: <br/> | ||
| + | <math>p_1 = 1</math><br/> | ||
| + | <math>p_2 = p_3 = ... = p_8 = 0</math><br/> | ||
| + | Zeros: <br/> | ||
| + | <math>z^{8} - 1 = 0 </math><br/> | ||
| + | <math>z^{8} = e^{j2\pi } </math><br/> | ||
| + | <math>z = e^{j2\pi /8 } </math><br/> | ||
| + | Generalizing,<br/> | ||
| + | <math>z_k = e^{j2\pi k/8 }</math> for k = 0,1,2,...,7<br/> | ||
---- | ---- | ||
| − | |||
| − | Back to [[ | + | Q6.<br/> |
| + | a. <br/> | ||
| + | <math> | ||
| + | y[n]= \frac{1}{8} \left( x[n]-x[n-8] \right) +y[n-1] | ||
| + | </math> | ||
| + | |||
| + | Using z-transform,<br/> | ||
| + | <math> | ||
| + | Y(z) = \frac{1}{8} \left( X(z)-X(z)z^{-8} \right) + Y(z)z^{-1} | ||
| + | </math><br/> | ||
| + | <math> | ||
| + | Y(z) (1 - z^{-1}) = X(z) \frac{1}{8}(1 - z^{-8}) | ||
| + | </math><br/> | ||
| + | <math> | ||
| + | H(z) = \frac{Y(z)}{X(z)} = \frac{1}{8} \left( \frac{ 1 - z^{-8} } {1 - z^{-1}} \right) | ||
| + | </math><br/> | ||
| + | b. Same as Q5, part c. <br/> | ||
| + | c. | ||
| + | H(z) can be re-written as <br/> | ||
| + | <math> | ||
| + | H[z]=\frac{1}{8} \left( 1+z^{-1}+z^{-2}+z^{-3}+z^{-4}+z^{-5}+z^{-6}+z^{-7} \right) | ||
| + | </math> <br/> | ||
| + | Taking inverse Z-transform of H(z) - <br/> | ||
| + | <math> | ||
| + | h[n]=\frac{1}{8} \left( \delta[n]+\delta[n-1]+\delta[n-2]+\delta[n-3]+\delta[n-4]+\delta[n-5]+\delta[n-6]+\delta[n-7] \right) | ||
| + | </math> <br/> | ||
| + | |||
| + | This is a finite duration response.<br/> | ||
| + | |||
| + | ---- | ||
| + | |||
| + | Back to [[Hw7ECE438F10|HW7]] | ||
| + | |||
| + | Back to [[2010 Fall ECE 438 Boutin|ECE 438 Fall 2010]] | ||
| + | |||
| + | [[Category:2010_Fall_ECE_438_Boutin]] | ||
Latest revision as of 11:23, 30 October 2011
Solution to HW7
Q1.
Recall, the Discrete Fourier Transform is defined as follows -
Definition: let x[n] be a DT signal with Period N. Then,
$ X [k] = \sum_{k=0}^{N-1} x[n].e^{-j2\pi kn/N} $
$ x [n] = (1/N) \sum_{k=0}^{N-1} X[k].e^{j2\pi kn/N} $
What is the relation between the DFT and the Fourier series coefficients of continuous periodic function x[n]?
The DFT of a sampled signal x[n] of length N is directly proportional to the Fourier series coefficients of the continuous periodic version of x[n].
The DFT of the N samples comprising one period of x[n] equals N times the Fourier series coefficients.
Alternatively -
The fourier series coefficients of a periodic, bandlimited signal x are given by the DFT of one period of the samples of x, divided by N, where N is the DFT length and N is also the number of samples in each period of x.
Credit: Julius Smith III, stanford.edu
$ x_1[n]= e^{j \frac{2}{3} \pi n}; $
Function's period N = 3,
Using IDFT,
$ \begin{align} x_1[n] &= \frac{1}{3} \sum_{k=0}^{2} X[k].e^{j2\pi kn/3} \\ e^{j \frac{2}{3} \pi n} &= \frac{1}{3} \sum_{k=0}^{2} X[k].e^{j2\pi kn/3} \\ &= \frac{1}{3} \left[ X[0].e^{j0} + X[1].e^{j2\pi n/3} + X[2].e^{j2\pi n(2/3)} \right] \\ &= \frac{1}{3} \left[ X[0] + X[1].e^{j2\pi n/3} + X[2].e^{j4\pi n/3} \right] \end{align} $
For the two sides to be equal,
X[0] = 0
X[1] = 3
X[2] = 0
Plugging in we can verify,
$ \begin{align} e^{j \frac{2}{3} \pi n} &= \frac{1}{3} \left[ 0 + 3.e^{j2\pi n/3} + 0 \right]\\ e^{j \frac{2}{3} \pi n} &= \frac{1}{3} 3.e^{j2\pi n/3} \\ e^{j \frac{2}{3} \pi n} &= e^{j \frac{2}{3} \pi n} \end{align} $
So our three selected values for X[k] are correct. Thus
$ X[k] = \begin{cases} 3, & k = 1 \\ 0, & \mbox{else} \end{cases} $
$ x_2[n]= e^{j \frac{2}{\sqrt{3}} \pi n}; $
Function x2[n] is aperiodic. Let's see why -
Assume x2[n] is periodic, then
$ e^{j \frac{2}{\sqrt{3}} \pi n} = e^{j \frac{2}{\sqrt{3}} \pi (n + N)} $ for function to be periodic, where N is an integer
$ e^{j \frac{2}{\sqrt{3}} \pi n} = e^{j \frac{2}{\sqrt{3}} \pi n}e^{j \frac{2}{\sqrt{3}} \pi N} $
$ e^{j \frac{2}{\sqrt{3}} \pi n} = e^{j \frac{2}{\sqrt{3}} \pi n}.(1) $
$ e^{j \frac{2}{\sqrt{3}} \pi N} = 1 $
For this to be true -
$ j \frac{2}{\sqrt{3}} \pi N = j 2\pi n, $ where n is an integer
$ N = n\sqrt{3} $
N is not an integer and this contradicts our assumption, proving that it cannot be true.
Thus, x_2[n] is aperiodic and we cannot apply the DFT.
$ x_3[n]= e^{j \frac{4}{3} \pi n}; $
Function's period N = 3,
Using IDFT,
$ \begin{align} x_3[n] &= \frac{1}{3} \sum_{k=0}^{2} X[k].e^{j2\pi kn/3} \\ e^{j \frac{4}{3} \pi n} &= \frac{1}{3} \sum_{k=0}^{2} X[k].e^{j2\pi kn/3} \\ &= \frac{1}{3} \left[ X[0].e^{j0} + X[1].e^{j2\pi n/3} + X[2].e^{j2\pi n(2/3)} \right] \\ &= \frac{1}{3} \left[ X[0] + X[1].e^{j2\pi n/3} + X[2].e^{j4\pi n/3} \right] \end{align} $
For the two sides to be equal,
X[0] = 0
X[1] = 0
X[2] = 3
$ X[k] = \begin{cases} 3, & k = 2 \\ 0, & \mbox{else} \end{cases} $
$ x_4[n]= e^{j \frac{2}{1000} \pi n}; $ Function's period N = 1000,
Using IDFT,
$ \begin{align} x_4[n] &= \frac{1}{1000} \sum_{k=0}^{999} X[k].e^{j2\pi kn/1000} \\ e^{j \frac{2}{1000} \pi n} &= \frac{1}{1000} \sum_{k=0}^{999} X[k].e^{j2\pi kn/1000} \\ &= \frac{1}{1000} \left[ X[0].e^{j0} + X[1].e^{j2\pi n/1000} + X[2].e^{j2\pi n(2/1000)} + ... \right] \\ &= \frac{1}{1000} \left[ X[0] + X[1].e^{j2\pi n/1000} + X[2].e^{j4\pi n/1000} + ... \right] \end{align} $
For the two sides to be equal,
X[0] = 0
X[1] = 1000
X[2] = 0
$ X[k] = \begin{cases} 1000, & k = 1 \\ 0, & \mbox{else} \end{cases} $
$ x_5[n]= e^{-j \frac{2}{1000} \pi n}; $ Function's period N = 1000,
$ \begin{align} x_5[n]&= e^{-j \frac{2}{1000} \pi n}.1 \\ &= e^{-j \frac{2}{1000} \pi n}.e^{-j 2\pi n} \\ &= e^{j 2\pi n(1 - (1/1000))} \\ &= e^{j 2\pi n\frac{999}{1000} } \\ \end{align} $
Using IDFT,
$ \begin{align} x_5[n] &= \frac{1}{1000} \sum_{k=0}^{999} X[k].e^{j2\pi kn/1000} \\ e^{j 2\pi n\frac{999}{1000}} &= \frac{1}{1000} \sum_{k=0}^{999} X[k].e^{j2\pi kn/1000} \\ &= \frac{1}{1000} \left[ X[0].e^{j0} + X[1].e^{j2\pi n/1000} + X[2].e^{j2\pi n(2/1000)}+ ... + X[999].e^{j2\pi n(999/1000)} \right] \\ &= \frac{1}{1000} \left[ X[0] + X[1].e^{j2\pi n/1000} + X[2].e^{j2\pi n (2/1000)} + ... + X[999].e^{j2\pi n(999/1000)} \right] \\ \end{align} $
For the two sides to be equal,
X[0] = 0
X[1] = 0
X[2] = 0
X[999] = 1000
$ X[k] = \begin{cases} 1000, & k = 999 \\ 0, & \mbox{else} \end{cases} $
$ \begin{align} x_6[n] &= \cos\left( \frac{2}{1000} \pi n\right) \\ &= \frac{1}{2}\left( e^{j\frac{2\pi n}{1000}} + e^{-j\frac{2\pi n}{1000}} \right) \\ &= \frac{1}{2} (x_4[n] + x_5[n]) \\ \end{align} $
We have an additional (1/2) to factor into the final coefficients, giving us -
$ X[k] = \begin{cases} 500, & k = 1 \\ 500, & k = 999 \\ 0, & \mbox{else} \end{cases} $
$ \begin{align} x_7[n] &= \cos^2\left( \frac{2}{1000} \pi n\right) \\ &= \left[ \frac{1}{2}\left( e^{j\frac{2\pi n}{1000}} + e^{-j\frac{2\pi n}{1000}} \right)\right]^2 \\ &= \frac{1}{4}\left( e^{j\frac{4\pi n}{1000}} + 2 + e^{-j\frac{4\pi n}{1000}} \right) \\ &= \frac{1}{4}\left( 2 + e^{j2\pi n\frac{2}{1000}} + e^{-j2\pi n\frac{2}{1000}}e^{-j2\pi n} \right) \\ &= \frac{1}{4}\left( 2 + e^{j2\pi n\frac{2}{1000}} + e^{j2\pi n\frac{998}{1000}} \right) \\ \end{align} $
Function's period N = 1000,
Using IDFT,
$ \begin{align} x_7[n] &= \frac{1}{1000} \sum_{k=0}^{999} X[k].e^{j2\pi kn/1000} \\ &= \frac{1}{1000} \left[ X[0] + X[1].e^{j2\pi n/1000} + X[2].e^{j2\pi n(2/1000)} + ... X[998].e^{j2\pi n(998/1000)} + X[999].e^{j2\pi n(999/1000)} \right] \\ \end{align} $
Comparing LHS and RHS,
X[0] = 500
X[1] = 0
X[2] = 250
...
X[998] = 250
X[999] = 0
$ X[k] = \begin{cases} 500, & k = 0 \\ 250, & k = 2 \\ 250, & k = 998 \\ 0, & \mbox{else} \end{cases} $
$ \begin{align} x_8[n]= (-j)^n \\ &= (e^{-j \pi /2})^n \\ &= e^{-j \pi n/2} \\ &= e^{-j \pi n/2}e^{j 2\pi} \\ &= e^{j 2\pi (3n/4)} \\ \end{align} $
Function's period N = 4,
Using IDFT,
$ \begin{align} x_8[n] &= \frac{1}{4} \sum_{k=0}^{3} X[k].e^{j2\pi kn/4} \\ e^{j 2\pi (3n/4)} &= \frac{1}{4} \sum_{k=0}^{3} X[k].e^{j2\pi kn/4} \\ &= \frac{1}{4} \left[ X[0].e^{j0} + X[1].e^{j2\pi n/4} + X[2].e^{j2\pi n(2/4)} + X[3].e^{j2\pi n(3/4)}\right] \\ &= \frac{1}{4} \left[ X[0] + X[1].e^{j\pi n/2} + X[2].e^{j\pi n} + X[3].e^{j\pi n(3/2)} \right] \end{align} $
For the two sides to be equal,
X[0] = 0
X[1] = 0
X[2] = 0
X[3] = 4
$ X[k] = \begin{cases} 4, & k = 3 \\ 0, & \mbox{else} \end{cases} $
Q2.
$ y_1[n]= \frac{x[n]+x[n-1]}{2} $
Applying Z-transform on both sides and grouping terms, we can obtain the transfer function
$ \begin{align} Y_1[z]&= \frac{X[z]+X[z].z^{-1}}{2} \\ \frac{Y_1[z]}{X[z]}&= \frac{1+z^{-1}}{2} \\ H_1[z] &= \frac{1+z^{-1}}{2} \\ \end{align} $
Frequency Response H_1(ω),
$ \begin{align} H_1[e^{j\omega }] &= \frac{1+e^{-j\omega }}{2} \\ &= e^{-j\frac{\omega }{2}} \left( \frac{e^{j\frac{\omega }{2}}+e^{-j\frac{\omega }{2}}}{2} \right) \\ &= e^{-j\frac{\omega }{2}} cos \left( \frac{\omega }{2} \right) \\ \end{align} $
$ y_2[n]= \frac{x[n]-x[n-1]}{2} $
Applying Z-transform on both sides and grouping terms, we can obtain the transfer function
$ \begin{align} Y_2[z]&= \frac{X[z]-X[z].z^{-1}}{2} \\ \frac{Y_2[z]}{X[z]}&= \frac{1-z^{-1}}{2} \\ H_2[z] &= \frac{1-z^{-1}}{2} \\ \end{align} $
Frequency Response H_2(ω),
$ \begin{align} H_2[e^{j\omega }] &= \frac{1-e^{-j\omega }}{2} \\ &= e^{-j\frac{\omega }{2}} \left( \frac{e^{j\frac{\omega }{2}}-e^{-j\frac{\omega }{2}}}{2} \right) \\ &= je^{-j\frac{\omega }{2}} \left( \frac{e^{j\frac{\omega }{2}}-e^{-j\frac{\omega }{2}}}{2j} \right) \\ &= je^{-j\frac{\omega }{2}} sin \left( \frac{\omega }{2} \right) \\ \end{align} $
$ y_3[n]= \frac{x[n+1]+x[n]+x[n-1]}{3} $
Applying Z-transform on both sides and grouping terms, we can obtain the transfer function
$ \begin{align} Y_3[z]&= \frac{X[z].z+ X[z] + X[z].z^{-1}}{3} \\ \frac{Y_3[z]}{X[z]}&= \frac{z(1+z^{-1} + z^{-2})}{3} \\ H_3[z] &= \frac{1+z^{-1} + z^{-2}}{3z^{-1}} \\ \end{align} $
Frequency Response H_3(ω),
$ \begin{align} H_3[e^{j\omega }] &= \frac{1+e^{-j\omega }+e^{-j2\omega }}{3e^{-j\omega }} \\ &= \frac{e^{-j\omega }+e^{-j\omega }(e^{j\omega } + e^{-j\omega })}{3e^{-j\omega }} \\ &= \frac{e^{-j\omega }(1+2cos(\omega ))}{3e^{-j\omega }} \\ &= \frac{1+2cos(\omega )}{3} \\ \end{align} $
$ y_4[n]= \frac{x[n+1]-2 x[n]+x[n-1]}{4}. $
Applying Z-transform on both sides and grouping terms, we can obtain the transfer function
$ \begin{align} Y_4[z]&= \frac{X[z].z- 2X[z] + X[z].z^{-1}}{4} \\ \frac{Y_3[z]}{X[z]}&= \frac{z(1-2z^{-1} + z^{-2})}{4} \\ H_3[z] &= \frac{1-2z^{-1} + z^{-2}}{4z^{-1}} \\ \end{align} $
Frequency Response H_3(ω),
$ \begin{align} H_4[e^{j\omega }] &= \frac{1-2e^{-j\omega }+e^{-j2\omega }}{4e^{-j\omega }} \\ &= \frac{-2e^{-j\omega }+ 2 e^{-j\omega }\frac{(e^{j\omega } + e^{-j\omega })}{2}}{4e^{-j\omega }} \\ &= \frac{2e^{-j\omega }(cos(\omega )-1)}{4e^{-j\omega }} \\ &= \frac{cos(\omega )-1}{2} \\ \end{align} $
Q3.
a. Substituting values directly would yield the following -
$ \begin{align} n &= ...\text{ -3, -2, -1, 0, 1, 2, 3, 4, 5} ...\\ y\left[n\right] &= ...\text{ 0, -2, -4, -1, 2, -1, -4, -2, 0} ...\\ \end{align} $
b. h[n] = ?
Substitute x[n] = δ[n] to obtain y[n] = h[n],
h[n] = δ[n] + 2δ[n − 1] + δ[n − 2]
Now x[n] = -2δ[n + 2] + δ[n] - 2δ[n − 2]
$ \begin{align} y[n] &= x[n] * h[n] \\ &= (-2\delta[n+2] + \delta[n] - 2\delta[n-2]) * (\delta[n] + 2\delta[n-1] + \delta[n-2]) \\ &= -2\delta[n+2] - 4\delta[n+1] -2\delta[n] + \delta[n] + 2\delta[n-1] + \delta[n-2] - 2\delta[n-2] - 4\delta[n-3] - 2\delta[n-4] \\ &= -2\delta[n+2] - 4\delta[n+1] - \delta[n] + 2\delta[n-1] - \delta[n-2] - 4\delta[n-3] - 2\delta[n-4] \\ \end{align} $
c. x[n] = ejωn
(i)
$ \begin{align} y[n] &= e^{j\omega n} + 2 e^{j\omega (n-1)} + e^{j\omega (n-2)} \\ &= e^{j\omega n}(1 + 2 e^{-j\omega } + e^{-2j\omega }) \\ H(e^{j\omega}) &= 1 + 2 e^{-j\omega } + e^{-2j\omega } \\ \end{align} $
(ii)
h[n] = δ[n] + 2δ[n − 1] + δ[n − 2]
H(ejω) = 1 + 2 e − jω + e − 2jω
(i) and (ii) are the same.
d. x[n] = -2δ[n + 2] + δ[n] - 2δ[n − 2]
X(ejω) = -2 e2jω + 1 - 2e − 2jω
$ \begin{align} Y(e^{j\omega}) &= X(e^{j\omega})H(e^{j\omega}) \\ &= (-2e^{2j\omega } + 1 - 2e^{-2j\omega } ).(1 + 2e^{-j\omega } + e^{-2j\omega }) \\ &= -2e^{2j\omega } - 4e^{j\omega } - 2 + 1 + 2e^{-j\omega } + 2e^{-2j\omega } - 2e^{-2j\omega } - 4e^{-3j\omega } - 2e^{-4j\omega } \\ &= -2e^{2j\omega } - 4e^{j\omega } - 1 - 2e^{-j\omega } - e^{-2j\omega } - 4e^{-3j\omega } - 2e^{-4j\omega } \\ \\ \text{Using Inverse DTFT,} \\ y[n] &= -2\delta[n+2] - 4\delta[n+1] - \delta[n] + 2\delta[n-1] - \delta[n-2] - 4\delta[n-3] - 2\delta[n-4] \\ \end{align} $
All 3 approaches lead to the same y[n].
Q4. $ H(z)= \frac{1-\frac{1}{2}z^{-2}} {1-\frac{1}{\sqrt{2}} z^{-1} +\frac{1}{4} z^{-2}} $
- a. Sketch the locations of the poles and zeros.
$ \begin{align} H(z) &= \frac{1-\frac{1}{2}z^{-2}}{1-\frac{1}{\sqrt{2}} z^{-1} +\frac{1}{4} z^{-2}} \\ H(z) &= \frac{(z+\frac{1}{\sqrt{2}})(z-\frac{1}{\sqrt{2}})} { (z-(\frac{1}{2\sqrt{2}} + j\frac{1}{2\sqrt{2}}))(z-(\frac{1}{2\sqrt{2}} - j\frac{1}{2\sqrt{2}})) } \\ \end{align} $
Zeros:
$ z_1 = \frac{1}{\sqrt{2}}, z_2 = -\frac{1}{\sqrt{2}} $
Poles:
$ p_1 = \frac{1}{2\sqrt{2}} + j\frac{1}{2\sqrt{2}}, p_2 = \frac{1}{2\sqrt{2}} - j\frac{1}{2\sqrt{2}} $
- b. Determine the magnitude and phase of the frequency response H(ω), for
$ \omega = 0 $
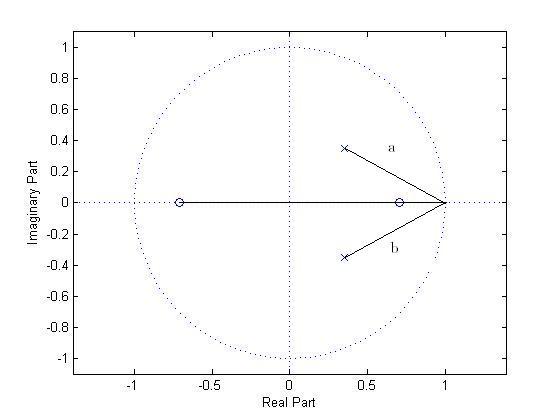 $ \left| H(e^{j\omega}) \right| = \left| H(e^{j0}) \right| = \left| H(z=1) \right| $
$ \left| H(e^{j\omega}) \right| = \left| H(e^{j0}) \right| = \left| H(z=1) \right| $
$ = \left| \frac{(1+\frac{1}{\sqrt{2}})(1-\frac{1}{\sqrt{2}})} { (1-(\frac{1}{2\sqrt{2}} + j\frac{1}{2\sqrt{2}}))(1-(\frac{1}{2\sqrt{2}} - j\frac{1}{2\sqrt{2}})) } \right| = 0.921 $
$ \angle H(e^{j0}) = \angle c + \angle d - \angle a - \angle b = 0 $
$ \omega =\frac{\pi}{4} $

$ = \left| \frac{(\frac{1}{\sqrt{2}} + j\frac{1}{\sqrt{2}}+\frac{1}{\sqrt{2}})(\frac{1}{\sqrt{2}} + j\frac{1}{\sqrt{2}}-\frac{1}{\sqrt{2}})} { (\frac{1}{\sqrt{2}} + j\frac{1}{\sqrt{2}}-(\frac{1}{2\sqrt{2}} + j\frac{1}{2\sqrt{2}}))(\frac{1}{\sqrt{2}} + j\frac{1}{\sqrt{2}}-(\frac{1}{2\sqrt{2}} - j\frac{1}{2\sqrt{2}})) } \right| = 2 $
$ \angle H(e^{j\frac{\pi}{4}}) = \angle c + \angle d - \angle a - \angle b = \frac{\pi}{2} + arctan^{-1} \left( \frac{\frac{1}{\sqrt{2}}}{\sqrt{2}} \right) - \frac{\pi}{4} - arctan^{-1} \left( \frac{\frac{1}{\sqrt{2}} + \frac{1}{2\sqrt{2}}}{1-\sqrt{2}-\frac{1}{2\sqrt{2}}} \right) = 0 $
$ \omega =\frac{\pi}{2} $
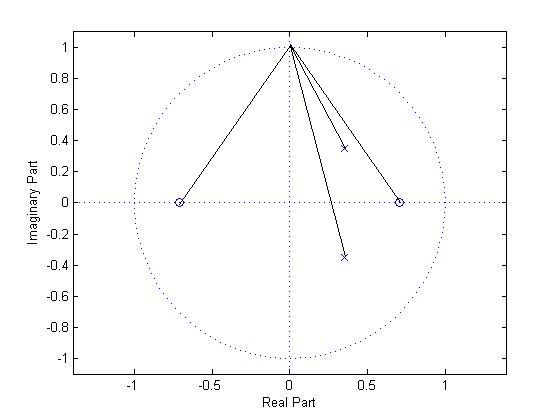 $ \left| H(e^{j\omega}) \right| = \left| H(e^{j\frac{\pi}{2}}) \right| = \left| H(z=j) \right| $
$ \left| H(e^{j\omega}) \right| = \left| H(e^{j\frac{\pi}{2}}) \right| = \left| H(z=j) \right| $
$ = \left| \frac{(j+\frac{1}{\sqrt{2}})(j-\frac{1}{\sqrt{2}})} { (j-(\frac{1}{2\sqrt{2}} + j\frac{1}{2\sqrt{2}}))(j-(\frac{1}{2\sqrt{2}} - j\frac{1}{2\sqrt{2}})) } \right| = 1.455 $
$ \angle H(e^{j\frac{\pi}{2}}) = (\angle c + \angle d) - \angle a - \angle b = (\pi) - (arctan^{-1}\left( \frac{1-\frac{1}{2\sqrt{2}}}{\frac{-1}{\sqrt{2}}} \right) + \pi) - (arctan^{-1}\left( \frac{1+\frac{1}{2\sqrt{2}}}{1-\frac{1}{\sqrt{2}}} \right) + \pi) = -0.7563 $
$ \omega =\frac{3\pi}{4} $

$ = \left| \frac{(\frac{-1}{\sqrt{2}} + j\frac{1}{\sqrt{2}}+\frac{1}{\sqrt{2}})(\frac{-1}{\sqrt{2}} + j\frac{1}{\sqrt{2}}-\frac{1}{\sqrt{2}})} { (\frac{-1}{\sqrt{2}} + j\frac{1}{\sqrt{2}}-(\frac{1}{2\sqrt{2}} + j\frac{1}{2\sqrt{2}}))(\frac{-1}{\sqrt{2}} + j\frac{1}{\sqrt{2}}-(\frac{1}{2\sqrt{2}} - j\frac{1}{2\sqrt{2}})) } \right| = \frac{2}{3} $
$ \angle H(e^{j\frac{3\pi}{4}}) = \angle c + \angle d - \angle a - \angle b = (arctan^{-1}\left( \frac{\frac{1}{\sqrt{2}}}{\frac{-2}{\sqrt{2}}} \right) + \pi) + \frac{\pi}{2} - (arctan^{-1}\left( \frac{\frac{1}{\sqrt{2}} - \frac{1}{2\sqrt{2}}}{\frac{1}{\sqrt{2}} - \frac{1}{2\sqrt{2}}} \right) + \pi) + \frac{3\pi}{4} = -0.9273 $
$ \omega =\pi $

$ = \left| \frac{(-1+\frac{1}{\sqrt{2}})(-1-\frac{1}{\sqrt{2}})} { (-1-(\frac{1}{2\sqrt{2}} + j\frac{1}{2\sqrt{2}}))(-1-(\frac{1}{2\sqrt{2}} - j\frac{1}{2\sqrt{2}})) } \right| = 0.255 $
$ \angle H(e^{j\pi}) = (\angle c + \angle d) - \angle a - \angle b = 2\pi - 0 - 0 = 2\pi $
c. Is the system stable? Explain why or why not?
The system is causal and the ROC extends outwards from the outermost pole since |$ p_1 $| = |$ p_2 $| < 1 and this ROC contains the unit circle. Therefore the system is stable.
d. Find the difference equation for y[n] in terms of x[n], corresponding to this transfer function H(z).
$ H(z) = \frac{Y(z)}{X(z)} = \frac{1-\frac{1}{2}z^{-2}}{1-\frac{1}{\sqrt{2}} z^{-1} +\frac{1}{4} z^{-2}} $
$ Y(z)(1-\frac{1}{\sqrt{2}} z^{-1} +\frac{1}{4} z^{-2}) = X(z)(1-\frac{1}{2}z^{-2}) $
Taking inverse,
$ y[n]-\frac{1}{\sqrt{2}}y[n-1] + \frac{1}{4}y[n-2] = x[n] - \frac{1}{2}x[n-2] $
$ y[n] = x[n] - \frac{1}{2}x[n-2] +\frac{1}{\sqrt{2}}y[n-1] - \frac{1}{4}y[n-2] $
Q5.
$ y[n]=\frac{1}{8} \left( x[n]+x[n-1]+x[n-2]+x[n-3]+x[n-4]+x[n-5]+x[n-6]+x[n-7]\right) $
a.
$ h[n]=\frac{1}{8} \left( \delta[n]+\delta[n-1]+\delta[n-2]+\delta[n-3]+\delta[n-4]+\delta[n-5]+\delta[n-6]+\delta[n-7] \right) $
This is a finite duration response.
b.
$ H[z]=\frac{1}{8} \left( 1+z^{-1}+z^{-2}+z^{-3}+z^{-4}+z^{-5}+z^{-6}+z^{-7} \right) $
$ H[z]=\frac{1}{8} \left( \frac{1-z^{-8}}{1-z^{-1}} \right) $
c.
$ H[z]=\frac{1}{8} \left( \frac{z^{8}-1}{z^{7}(z-1)} \right) $
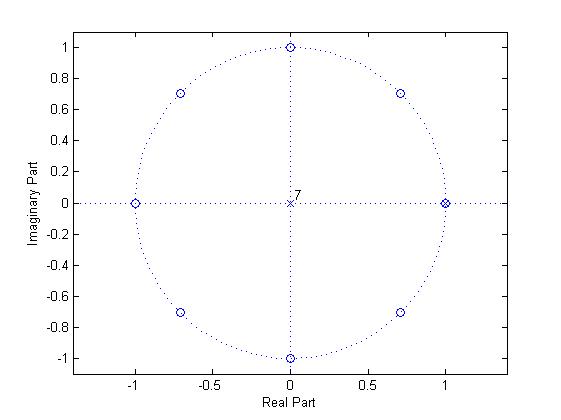
Poles:
$ p_1 = 1 $
$ p_2 = p_3 = ... = p_8 = 0 $
Zeros:
$ z^{8} - 1 = 0 $
$ z^{8} = e^{j2\pi } $
$ z = e^{j2\pi /8 } $
Generalizing,
$ z_k = e^{j2\pi k/8 } $ for k = 0,1,2,...,7
Q6.
a.
$ y[n]= \frac{1}{8} \left( x[n]-x[n-8] \right) +y[n-1] $
Using z-transform,
$ Y(z) = \frac{1}{8} \left( X(z)-X(z)z^{-8} \right) + Y(z)z^{-1} $
$ Y(z) (1 - z^{-1}) = X(z) \frac{1}{8}(1 - z^{-8}) $
$ H(z) = \frac{Y(z)}{X(z)} = \frac{1}{8} \left( \frac{ 1 - z^{-8} } {1 - z^{-1}} \right) $
b. Same as Q5, part c.
c.
H(z) can be re-written as
$ H[z]=\frac{1}{8} \left( 1+z^{-1}+z^{-2}+z^{-3}+z^{-4}+z^{-5}+z^{-6}+z^{-7} \right) $
Taking inverse Z-transform of H(z) -
$ h[n]=\frac{1}{8} \left( \delta[n]+\delta[n-1]+\delta[n-2]+\delta[n-3]+\delta[n-4]+\delta[n-5]+\delta[n-6]+\delta[n-7] \right) $
This is a finite duration response.
Back to HW7
Back to ECE 438 Fall 2010


