Difference between revisions of "Cantor, sets, and continua MA375S12Walther" - Rhea

Revision as of 14:38, 25 April 2012

The Cantor set is a famous set first constructed by Georg Cantor in 1883. It is simply a subset of the interval [0, 1], but the set has some very interesting properties. We will first describe how to construct this set, and then prove some interesting properties of the set.

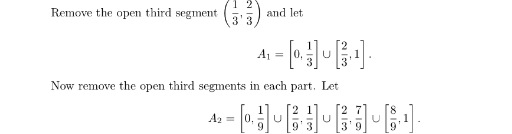Continue in this way always removing the middle third of each segment to get A3,A4, . . .. Note that A1 ⊇ A2 ⊇ A3 ⊇ · · · . And for each k ∈ N, Ak is the union of 2k closed intervals, each of length 3−k. Let C = ∩1 i=1Ai. Then C is the Cantor set. Now we will prove some interesting properties of C.

The term fractal is used to describe objects that cannot be effectively described using normal Euclidean geometry. The word “fractal” is somewhat synonymous with the word “broken”. Below is an example of a well-known fractal object called the Cantor Set. The Cantor Set is created by the following algorithm: Start with the closed interval [0,1]. Call this set ��, or the 0th (initial) set.

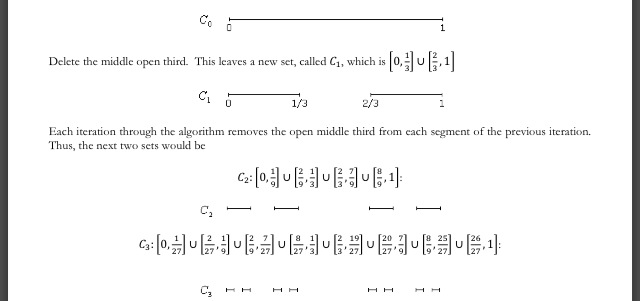You can see the set becomes more sparse the more iterations we perform. The Cantor Set is defined to be the set of the points that remain as the number of iterations tends to infinity. If the Cantor Set was composed of discrete points, then every point would be “separate” from every other point. If this was true, we could enclose any point in the Cantor Set inside a very tiny circle of radius � such that the circle encloses that one point only. However, we can demonstrate that for any such radius, there will always be infinitely many other points from the Cantor Set within this circle. For example, we agreed that any point that is the endpoint of an interval at any iteration remains. So let’s pick x=1/3. This point will never be deleted and is a member of the Cantor Set. So now let’s pick a small value for delta,say, delta = 1/100 Our claim is that the point x=1/3

is

[Category:MA375Spring2012Walther]