| Line 4: | Line 4: | ||
<center>[[Image:Point_charge.png]]</center> | <center>[[Image:Point_charge.png]]</center> | ||
| + | |||
| + | The simple equation for finding the charge would be | ||
[[ Walther MA271 Fall2020 topic14 | Back to Feynman Integrals]] | [[ Walther MA271 Fall2020 topic14 | Back to Feynman Integrals]] | ||
[[Category:MA271Fall2020Walther]] | [[Category:MA271Fall2020Walther]] | ||
Revision as of 17:43, 4 December 2020
Application of Leibniz's Rule in Electrostatics
From what we've seen about the Feynman/Leibniz rule of integration, we now have an additional method of integrating that will be able to find us solutions for various complex integrals that would have been much more difficult to solve before. This, however, doesn't apply solely to the field of mathematics. Since many areas of study utilize the helpfulness of integration and calculating values through derivates, it can be assumed that they will also benefit a lot from the Feynman technique. Physics in particular has a lot of use for these concepts, with velocity and acceleration being important aspects of the field, and its use continues throughout the rest of the topics. For example, one of these topics is electrostatics, which deals with the idea of calculation charge on a particle.
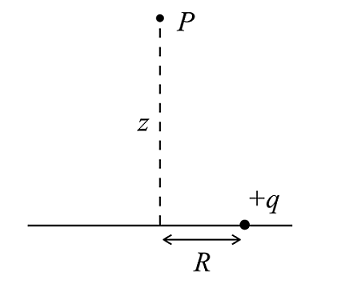
The simple equation for finding the charge would be

