(→Synchronous Demodulation (with phase error) in the Frequency DomainAgain) |
|||
| (11 intermediate revisions by the same user not shown) | |||
| Line 32: | Line 32: | ||
[[Image:aaa_ECE301Fall2008mboutin.jpg]] | [[Image:aaa_ECE301Fall2008mboutin.jpg]] | ||
[[Image:asd_ECE301Fall2008mboutin.jpg]] | [[Image:asd_ECE301Fall2008mboutin.jpg]] | ||
| + | |||
| + | == Synchronous Demodulation (with phase error) in the Frequency DomainAgain == | ||
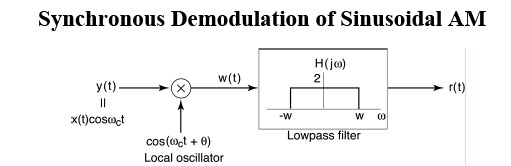
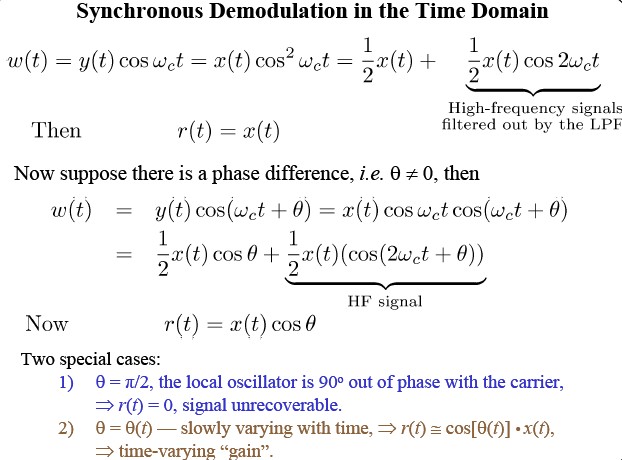
| + | Demodulating signal has phase difference θw.r.t.the modulating signal | ||
| + | |||
| + | <math>cos(\omega_{C}t+\theta)= \frac{1}{2}e^{j\theta}e^{j\omega_{c}t}+\frac{1}{2}e^{-j\theta}e^{-j\omega_{c}t}</math> | ||
| + | |||
| + | fourier ====> | ||
| + | |||
| + | [[Image:aaaa_ECE301Fall2008mboutin.jpg]] | ||
| + | [[Image:aaaaa_ECE301Fall2008mboutin.jpg]] | ||
Latest revision as of 20:00, 17 November 2008
Contents
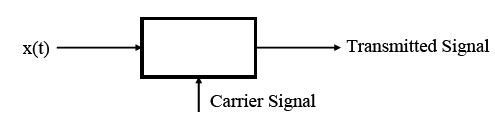
the concept of modulation
•More efficient to transmit E&M signals at higher frequencies
•Transmitting multiple signals through the same medium using different carriers
•Transmitting through “channels” with limited passbands
•Others...
How?
•Manymethods
•Focus here for the most part on Amplitude Modulation (AM)
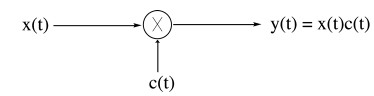
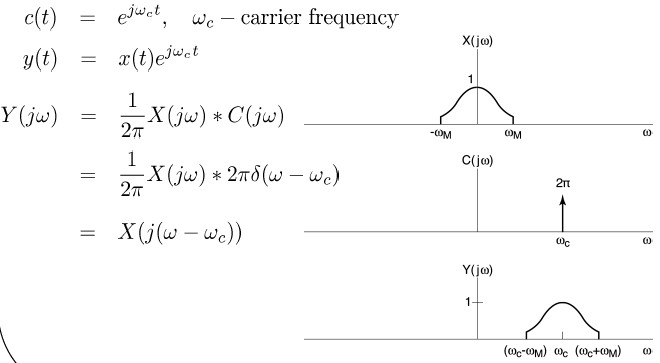
Amplitude Modulatioin of a Complex Exponential Carrier
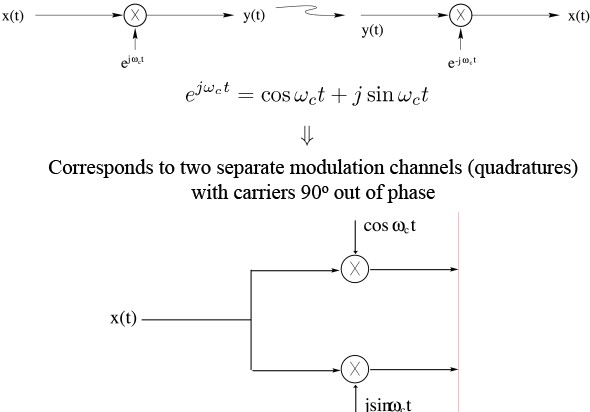
Demodulation of Complex Exponential AM
Sinusoidal AM
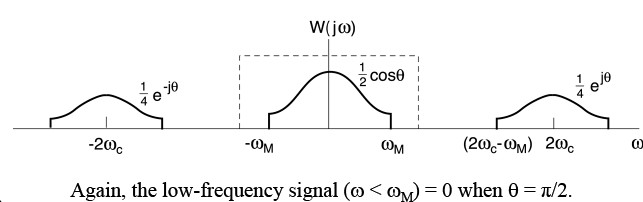
Synchronous Demodulation (with phase error) in the Frequency DomainAgain
Demodulating signal has phase difference θw.r.t.the modulating signal
$ cos(\omega_{C}t+\theta)= \frac{1}{2}e^{j\theta}e^{j\omega_{c}t}+\frac{1}{2}e^{-j\theta}e^{-j\omega_{c}t} $
fourier ====>